Geometry

Hong Kong
Stage 1 - Stage 3
Alternate Angles
Lesson
Alternate angles
Alternate angles in parallel lines are equal.
A pair of angles are said to be alternate if
- the angles are made from two parallel lines and 1 transversal line (line that crosses the parallel lines)
- the angles are not adjacent (not next to each other)
- the two angles do not share a vertex
- the two angles share a common arm, with angles appearing on opposite sides of that arm.
In these images, the alternate angles are marked.
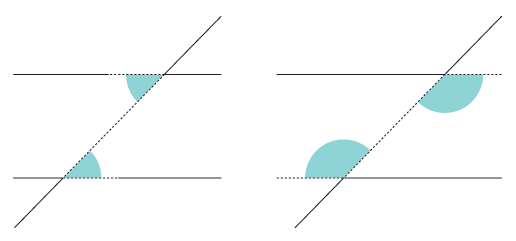
We can use a picture like this to help us remember this rule.
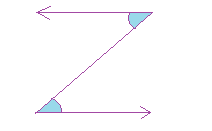
Alternate Angles
Alternate angles in parallel lines are equal.
(To help remember this is sometimes referred to as the z rule).
This interactive shows you how alternate angles are equal. You can move the parallel lines, and the transversal, by moving the blue points. This will create different angles, and you can view the angles one at a time or both together.
Worked Examples
Question 1
Calculate $x$x giving reasons for your answer.
Loading video...
Question 2
Using only alternate angles, calculate $x$x
Loading video...