Grade 10

Solve contextual problems in right triangles

Lesson

Practice

Lesson

We have already seen what the trigonometric ratios are,

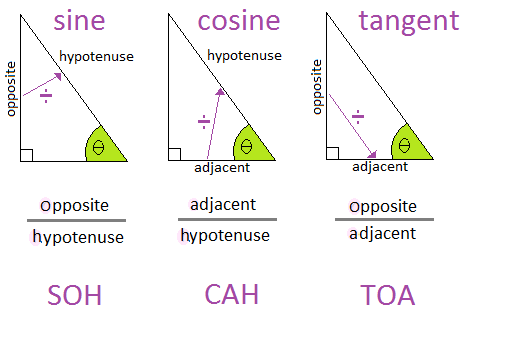
We know how to calculate with them, and how to find the length of unknown sides of right-angled triangles with them.

Find the size of an unknown side

Construct a triangle that represents the given situation, labelling all known values
Label the sides as O, A or H with respect to the position of the known angle
Identify the appropriate trigonometric ratio that applies [either sine (sin), cosine (cos) or tangent (tan)]
Using algebra, solve the equation for the angle, (write the rule, fill in what you know, then rearrange to solve for the unknown side length)
Reflect and check (do a quick check on your calculator to confirm your answer is correct)

We have also seen how to use the trigonometric ratios to find the size of unknown angles. To do this we need any $2$2 of the side lengths.

Find the Size of an unknown angle

Construct a triangle that represents the given situation, labelling all known values
Label the sides as O, A or H with respect to the position of the angle you want to find
Identify the appropriate trigonometric ratio that applies [either sine (sin), cosine (cos) or tangent (tan)]
Using algebra, solve the equation for the angle, (write the rule, fill in what you know, then solve using inverse operations)
Reflect and check (do a quick check on your calculator to confirm your answer is correct)

Practice questions

Question 1

The person in the picture sights a paraglider above him.

If the angle the person is looking at is $a$a, find $a$a to two decimal places.

An angle is formed when a person sights a paraglider above him from his horizontal line of sight. A right-angled triangle is formed by the horizontal line of sight, the vertical distance between the person and the paraglider, and the actual distance between the person and the paraglider. The horizontal line of sight forms a $a$`a`º angle of elevation with the distance between the person and the paraglider. A vertical distance between the paraglider to the person's horizontal line of sight is $11$11 m which represents the opposite side of the angle if elevation. While the horizontal distance between them is $13$13 m which represent the adjacent side of the angle of elevation.

Question 2

If $d$d is the distance between the base of the wall and the base of the ladder, find $d$d to two decimal places.

A ladder $1.45$ -m long leans against a vertical brick wall. The base of the ladder makes an angle with the ground measuring $38^\circ$38°. Dotted lines highlight the right-angled triangle formed by the wall, the ground, and ladder. The length of the ladder represents the hypotenuse. Adjacent to the $38^\circ$38° angle is the distance from the base of the ladder to the base of the wall which labeled as $d$`d` m, indicating its length. Opposite to the $38^\circ$38° angle is the distance from the base of the wall up to the point where the ladder touches the wall.

Question 3

$Jack$ is standing at the tip of a tree's shadow and knows that the angle from the ground to the top of the tree is $34^\circ$34°.

If $Jack$ is standing $29$29 metres away from the base of the tree, what is the value of $h$h, the height of the tree to the nearest two decimal places?

Outcomes

10D.T2.03

Solve problems involving the measures of sides and angles in right triangles in real life applications, using the primary trigonometric ratios and the Pythagorean theorem.