
Congruence in Quadrilaterals
We've looked at congruent triangles and how to prove that triangles are congruent.
- SSS - all corresponding sides are equal.
- SAS - 2 pairs of corresponding sides are equal and the included angles are equal.
- AAS - 2 angles are equal and one pair of corresponding sides is equal.
- RHS - both triangles have right angles, hypotenuses are equal and one pair of corresponding sides are equal.
In this chapter, we are going to extend that knowledge and look at how we can join two congruent triangles to make a quadrilateral, and hence, how we can make deductions about these four-sided shapes. To do this, we need to look at the properties of some of these quadrilaterals. Here is a recap.
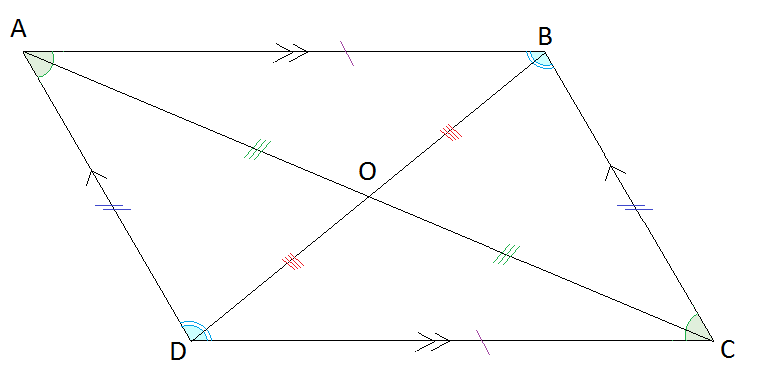
Parallelogram
- Opposite sides in a parallelogram are parallel
- Opposite angles in a parallelogram are equal
- Opposite sides in a parallelogram are equal
- Diagonals of a parallelogram bisect each other

Rectangle
- Opposite sides in a rectangle are parallel
- Opposite sides in a rectangle are equal
- Diagonals of a rectangle bisect each other
- Diagonals in a rectangle are equal
- All angles are right angles

Square
- All sides of a square are equal
- Opposite sides in a square are parallel
- Diagonals of a square are perpendicular to each other (cross at 90°)
- Diagonals of a square bisect the angles at the vertices (makes them 45°)
- Diagonals of a square bisect each other
- Diagonals of a square are equal
Rhombus

- Opposite angles of a rhombus are equal
- Opposite sides in a rhombus are parallel
- All sides of a rhombus are equal
- Diagonals of a rhombus bisect each other at 90 degrees
- Diagonals of a rhombus bisect corner angles
- Diagonals of a rhombus bisect each other

Trapezium
- An isosceles trapezium (trapezoid) has 2 pairs of adjacent angles equal
- A trapezium (trapezoid) has one pair of opposite sides parallel
- An isosceles trapezium (trapezoid) has one pair of opposites sides equal
- Diagonals of an isosceles trapezium (trapezoid) are equal
Kite

- A kite has 2 pairs of adjacent sides equal
- A kite has 1 pair of opposite angles equal
- The longest diagonal of a kite bisects the angles through which it passes
- Diagonals of a kite are perpendicular to each other
- The longest diagonal of a kite bisects the shorter diagonal
Now let's look through some examples to see this process in action.
Examples
Question 1
The following kite is formed by joining two congruent triangles. What type of triangles are they?
A) scalene B) isosceles C) equilateral
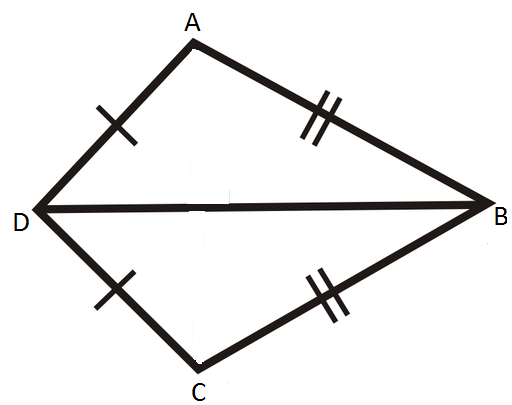
Think: What are the properties of these different types of triangles.
Do: The picture does not indicate that any of the side lengths are the same. So these triangles are scalene.
Question 2
$WXYZ$WXYZ is a rectangle. $\triangle WXZ$△WXZ is congruent to $\triangle XYW$△XYW.
a) Deduce the side that is equal in length to $WY$WY.
b) Then, what can we deduce about the diagonals of a rectangle?
Question 3
Two identical isosceles triangles are joined along the unequal edge. What kind of quadrilateral is formed?