Volume

Previously we discovered that for rectangular prisms the volume can be found by multiplying:

$\text{Base }\times\text{Width }\times\text{Height }$Base ×Width ×Height

A prism is defined as a solid geometric figure whose two end faces are similar, equal, straight and parallel.  Both of these are prisms...

Prisms occur very commonly in packaging of grocery items, and finding the volume of these contributes to the design, shape and size of packaging and product - and all of these affect the price that we pay!

To experiment with how the volume of a triangular prism is affected by its base and height, or to see how the volume of a rectangular prism is affected by its base, width and height, try the following mathlet. You can vary the dimensions by moving the sliders and expose the volume by checking the checkbox.

Volume of Any Prism

If the solid is a prism, its volume can be worked out by using the rule:

The base is the face that is extended throughout the height. Another way of identifying the base is finding the two faces that are identical and parallel to one another.  

So, in the case of a triangular prism, the volume would be found by: 

$\text{Area of the triangle }\times\text{height of the prism }$Area of the triangle ×height of the prism .

For a trapezium based prism, the volume will be the $\text{area of the trapezium }\times\text{height of the prism}$area of the trapezium ×height of the prism .

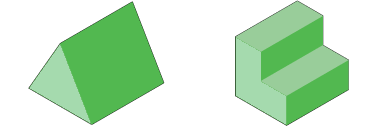
For the volume of a complex shaped prism like this we would find the $\text{area of the base (L shape) }\times\text{height of the prism }$area of the base (L shape) ×height of the prism .  

Practical example of Volumes

You are about to go camping for the first time and need to buy a tent. You visit the local store that sells camping gear and one tent immediately attracts your attention but it appears to be a little too small for your liking. When you ask one of the salesmen for a tent with a larger volume he points you to a tent that has a similar length but double the height and that he claims is the largest tent in store. When you ask another salesman he points you to a tent that has a similar height to your original choice but double the length and that he claims is the largest tent in store. Who is right? Can they both be right?

If you think back to the formula for the volume of a triangular prism, $\frac{1}{2}\times\text{base }\times\text{height }\times\text{length of prism }$12​×base ×height ×length of prism if the prism is laid out like a tent, you will notice that when the height doubles, so too will the volume; and when the length doubles, so too will the volume. So in the case of the salesmen, both of them could be right because both of the tents they point out have the same volume.

Worked Examples

QUESTION 1

Find the volume of the cube shown.

Reveal SolutionWatch video

QUESTION 2

Find the volume of the triangular prism shown.

Reveal SolutionWatch video

QUESTION 3