2.02 Polynomial functions
Polynomial curve sketching
Certain polynomials of degree $n$n can be factored into $n$n linear factors over the real number field.
For example the $4$4th degree polynomial $P\left(x\right)=2x^4-x^3-17x^2+16x+12$P(x)=2x4−x3−17x2+16x+12 can be re-expressed as $P\left(x\right)=\left(x+3\right)\left(2x+1\right)\left(x-2\right)^2$P(x)=(x+3)(2x+1)(x−2)2. Note that there are two distinct factors, the $\left(x+3\right)$(x+3) and $\left(2x+1\right)$(2x+1) and two equal factors, the $\left(x-2\right)$(x−2) appears twice.
A polynomial function $y=P\left(x\right)$y=P(x) that can be completely factored into its linear factors can be easily sketched. The function $y=\left(x+3\right)\left(2x+1\right)\left(x-2\right)^2$y=(x+3)(2x+1)(x−2)2 has roots immediately identifiable as $x=-3,-\frac{1}{2}$x=−3,−12 and $x=2$x=2.
Multiplicity
A root is said to have multiplicity $k$k if a linear factor occurs $k$k times in the polynomial function. For example the function of degree $6$6 given by $y=\left(x-1\right)\left(x+1\right)^2\left(x-2\right)^3$y=(x−1)(x+1)2(x−2)3 is said to have a root $x=1$x=1 of multiplicity $1$1 (a single root), another root of $x=-1$x=−1 of multiplicity $2$2 (a double root or two equal roots) and a root $x=2$x=2 of multiplicity $3$3 (a triple root or three equal roots). In effect that is $6$6 roots all together.
The key to understanding how a root's multiplicity effects the graph is given in the following statement:
The curve's shape near a root depends on the root's multiplicity.
Look closely at the sketch of $y=\left(x-1\right)\left(x+1\right)^2\left(x-2\right)^3$y=(x−1)(x+1)2(x−2)3

The curve approaching a root of multiplicity $1$1 will behave like a linear function across that root (see position B on graph). The curve approaching a root of multiplicity $2$2 will behave like a quadratic function across that root (see position A on graph). The curve approaching a root of multiplicity $3$3 will behave like a cubic function across that root (see position C on graph).
In general, the curve approaching a root of multiplicity $k$k will behave like the function $y=x^k$y=xk across that root.
There is also two other considerations that will help with the graphing of the function.
Firstly, note that this function is an even degree function, so at the left and right extremes it will move away from the $x$x - axis in the same direction. Odd degree functions move away in opposite directions.
Secondly, because the coefficient of the greatest power of the function is positive, the curve moves away from the $x$x - axis positively.
Thirdly, always check the position of the $y$y - intercept. For example, in the above sketch, at $x=0$x=0, $y=\left(0-1\right)\left(0+1\right)^2\left(0-2\right)^3=8$y=(0−1)(0+1)2(0−2)3=8 and this confirms the direction of the curve downward toward the first positive root.
In all this we need more mathematical tools to determine the positions of local turning points, but the important principles here allow us to understand the functions basic shape.
Worked examples
Question 1
The function $y=x\left(x+3\right)\left(x-2\right)$y=x(x+3)(x−2) has three roots $0,-3$0,−3 and $2$2 all of multiplicity $1$1. It is of degree $3$3 with a $y$y-intercept of $\left(0\right)\left(-3\right)\left(2\right)=0$(0)(−3)(2)=0. The shape is shown as graph $G2$G2 here.

Question 2
The function $y=-\left(x-1\right)\left(x+2\right)^2$y=−(x−1)(x+2)2 has a root of multiplicity $1$1 at $x=1$x=1 and a root of multiplicity $2$2 ($2$2 equal roots) at $x=-2$x=−2. The $y$y - intercept is $-\left(-1\right)\left(2\right)^2=4$−(−1)(2)2=4. The function is of odd degree, so its ends move off in different directions. Note that for large positive values of $x$x, the curve becomes very negative (See graph $G3$G3 below).

Question 3
The function $y=\frac{1}{2}\left(x-3\right)^2\left(x+1\right)^3$y=12(x−3)2(x+1)3 is of degree $5$5, with a double root at $x=3$x=3 and a triple root at $x=-1$x=−1. The y intercept is $4.5$4.5. The coefficient $\frac{1}{2}$12 at the front simply halves the size of every $y$y value, but note that there is no change at all in the position of the roots because half of zero is still zero (see graph $G4$G4 below).

Practice questions
Question 4
Consider the curve $y=\left(x+3\right)\left(x+2\right)\left(x-2\right)$y=(x+3)(x+2)(x−2).
Find the $x$x-value of the $x$x-intercept(s).
Find the $y$y-value of the $y$y-intercept(s).
Sketch a graph of the curve.
Loading Graph...
Question 5
Consider the curve $y=-\left(x-3\right)^2\left(x+1\right)\left(x-2\right)$y=−(x−3)2(x+1)(x−2).
Find the $x$x-value(s) of the $x$x-intercept(s).
Find the $y$y-value(s) of the $y$y-intercept(s).
Plot the graph of the curve.
Remember that for a curve of degree $n$n, we need $n+1$n+1 unique points.
Loading Graph...
Question 6
The graph of $y=P\left(x\right)$y=P(x) is shown. Plot the graph of $y=P\left(x+5\right)$y=P(x+5).
- Loading Graph...
Applications of polynomials
Worked examples
Question 7
Brooklyn Bridge is a cable stayed suspension bridge in New York City. Its construction was one of the industrial wonders of the modern world. The curved cable is parabolic and the approximate dimensions of the bridge within the two massive pylons are indicated in the diagram here:
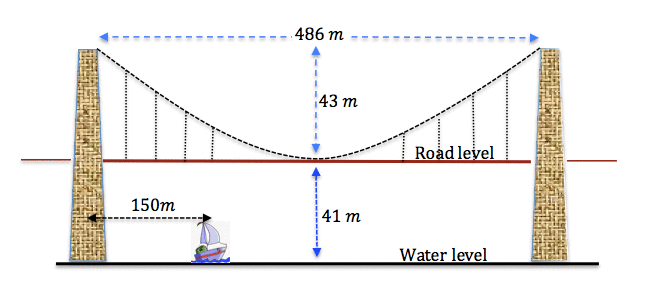
Suppose we have been asked to determine the height above sea-level of the cable at the point directly above a sailing ship that is $150$150 meters in from the left pylon as shown.
Think: We first need to model the suspension cable with a parabola. To do this we imagine an $x$x - axis at water level and a $y$y -axis through the least point of the cable, so that we can write coordinates of the least cable point as $\left(0,41\right)$(0,41).
Our parabola then has the form $y=ax^2+41$y=ax2+41, and we need to determine the leading coefficient $a$a.
To do this we need another point, and with a little thought, we can ascertain that the coordinate of the point where the cable ties to the top of the right pylon as $\left(243,84\right)$(243,84). This point must satisfy our equation and so:
Do:
| $84$84 | $=$= | $a\left(243\right)^2+41$a(243)2+41 |
| $59049a$59049a | $=$= | $43$43 |
| $a$a | $=$= | $\frac{43}{59049}$4359049 |
Reflect: The value of $a$a is extremely small (approximately $0.000728$0.000728) which makes sense considering that the length of the bridge is about ten times the height at road level.
This means that our cable parabola is given by $y=\left(\frac{43}{59049}\right)x^2+41$y=(4359049)x2+41.
If the ship is $150$150 m from the left pylon, then based on our coordinate system, the $x$x value of the water level point must be given by $x=-\left(243-150\right)=-97$x=−(243−150)=−97. Thus when we substitute this value into our equation we have:
$y=\left(\frac{43}{59049}\right)\left(-97\right)^2+41=47.852$y=(4359049)(−97)2+41=47.852
Hence the cable point directly above the ship is approximately $48$48 meters above sea-level.
Question 8
A cricket ball is fired vertically upwards by a machine so that at time $t$t seconds the height $h$h meters is given by $h=-4.9t^2+39.2t$h=−4.9t2+39.2t. Determine its maximum height.
Think: If we take the common factor $-4.9t$−4.9t out we see that $h=-4.9t\left(t-8\right)$h=−4.9t(t−8).
Do: We thus know by setting $h=0$h=0, that the time of flight is $8$8 seconds.
Because a graph of height versus time is an inverted parabola, we know that the maximum height is reached when $t=4$t=4 seconds.
At $t=4$t=4, $h=-4.9\left(4\right)^2+39.2\left(4\right)=78.4$h=−4.9(4)2+39.2(4)=78.4 meters
Question 9
A certain ordered listing of numbers have a sum, up to and including the $n$nth term, given by the formula $S_n=\frac{n}{2}\left(7n-1\right)$Sn=n2(7n−1).
So for example, the list up to the sixth term sums to $S_6=\frac{6}{2}\left(7\times6-1\right)=123$S6=62(7×6−1)=123.
We might ask how many numbers in the list must be added together so that the sum becomes $3348$3348?
Think: We can get a feel for the list by recognizing that $S_1=\frac{1}{2}\left(7\times1-1\right)=3$S1=12(7×1−1)=3, and so $3$3 must be the first term of the list.
Do: With $S_2=\frac{2}{2}\left(7\times2-1\right)=13$S2=22(7×2−1)=13 we know therefore that the second term is $10$10, so that $3+10=13$3+10=13. The third term could likewise be revealed as $17$17, and the fourth term $24$24 etc.
The question asks when does $S_n=\frac{n}{2}\left(7n-1\right)=3348$Sn=n2(7n−1)=3348? This is a quadratic equation that can be solved as follows:
| $\frac{n}{2}\left(7n-1\right)$n2(7n−1) | $=$= | $3348$3348 |
| $n\left(7n-1\right)$n(7n−1) | $=$= | $6696$6696 |
| $7n^2-n$7n2−n | $=$= | $6696$6696 |
| $7n^2-n-6696$7n2−n−6696 | $=$= | $0$0 |
| $\left(7n+216\right)\left(n-31\right)$(7n+216)(n−31) | $=$= | $0$0 |
| $n$n | $=$= | $31,-\frac{216}{7}$31,−2167 |
Reflect: Thus, since $n$n must be positive, $31$31 terms are required so that the sum is $3348$3348.
Practice questions
QUESTION 10
The sum of the series 1+2+3+...+$n$n is given by $S$S=$\frac{n\left(n+1\right)}{2}$n(n+1)2.
Find the sum of the first $32$32 integers.
Find the number of integers required for a sum of $15$15. (Let the number of integers be $n$n.)
QUESTION 11
Mae throws a stick vertically upwards. After $t$t seconds its height $h$h meters above the ground is given by the formula $h=25t-5t^2$h=25t−5t2.
At what time(s) will the stick be $30$30 meters above the ground?
The stick takes a total of $T$T seconds to hit the ground. Find the value of $T$T.
Can the stick ever reach a height of $36$36 meters?
Yes
ANo
B
QUESTION 12
The arch of a bridge is built so that its height above the horizontal road can be modeled by a parabola whose equation is $y=-\frac{1}{8}\left(x^2-400\right)$y=−18(x2−400), where $y$y represents the height of the arch above the road at distance $x$x meters from the center of the bridge.

Determine the peak height of the arch.
Pedestrians can climb along the arch up to a point that is horizontally halfway from the center of the bridge. State the possible $x$x values of this point on the bridge.
Write the two values on the same line separated by a comma.
At this point, how high up are the pedestrians? Give your answer correct to two decimal places.
For what values of $x$x does this model not work?
$x\le-20$x≤−20 and $x\ge20$x≥20
A$x<-20$x<−20 and $x>20$x>20
B$-20$−20 $<$< $x$x $<$< $20$20
C$x<0$x<0
D