7.08 Normal distribution

There are a lot of quantities in real life that exhibit natural variation, for example people's heights, IQ and weights. Even in unnatural processes there can be random errors that result in variation.

For example, consider a manufacturer which produces a chocolate bar with a weight of $50$50 g. It is impossible to make every chocolate bar weigh exactly $50$50 g. But if we weigh $100$100 chocolate bars we would expect the mean value to be very close to $50$50 g. We would also expect most of the measurements to be close to $50$50 g with roughly the same variation above and below $50$50 g, and relatively few measurements that differ significantly from $50$50 g.

If the distribution of chocolate bar weights was displayed in a column graph, the column graph would show a central peak with columns of decreasing height on each side of the mean.

If there could be a vast number of observations put into the column graph with very narrow column widths, the variety of observations would give a bell-shaped column graph that reflects the way in which the frequencies of the observations diminish for values increasingly distant from the mean.

A data set that is symmetrical and bell-shaped about the mean, is said to have a normal distribution.

Two important values are needed to graph or interpret a normal distribution: the mean and the standard deviation.

The mean (represented by the symbol "mu", $\mu$μ) is the location of the maximum density of observations and is where the curve is highest. The curve is symmetrical about the mean. In fact, in a normally distributed data set, the mean, median and mode all take the same value.

The spread of the normal distribution will depend on its variance or the standard deviation (represented by the symbol "sigma", $\sigma$σ) which is the square root of the variance. This will affect how wide and how high the normal distribution is when graphed.

If the standard deviation is low, the proportion of observations near the mean will be high and the curve will be high at the mean value. If the standard deviation is high, the proportion of observations near the mean is reduced and therefore the curve is not so high at the mean.

For example, this distribution curve comes from a data set that has a very small standard deviation, $\sigma=0.2$σ=0.2, and hence is clustered tightly around the mean:

whereas this normal distribution has a larger standard deviation, $\sigma=0.9$σ=0.9, and hence is spread out.

 

To see how a change in the mean and standard deviation affects the shape of the normal distribution curve, check out the following applet. By moving the sliders around you can vary the mean and standard deviation, and see how the bell curve changes as a result.

 

Practice questions

QUESTION 1

QUESTION 2

QUESTION 3

 

Empirical rule

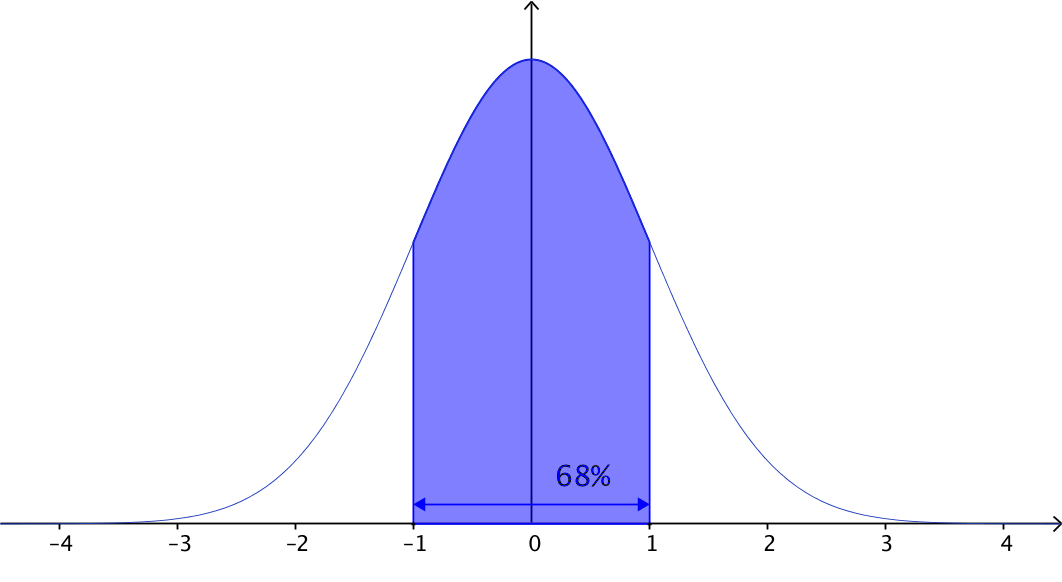
The empirical rule, also known as the $68$68-$95$95-$99.7$99.7$%$% rule, is a way of estimating the way that normally distributed data spreads out. These numbers correspond to the approximate proportion within one, two, and three standard deviations of the mean.

• Approximately $68%$68% of data values lie within $1$1 standard deviation of the mean:

• Approximately $95%$95% of data values lie within $2$2 standard deviations of the mean:

• Approximately $99.7%$99.7% of data values lie within $3$3 standard deviations of the mean:

A normal distribution is symmetrical, so we can use these basic values to find approximations of other regions. For example, as $95%$95% of data values lie within $2$2 standard deviations of the mean, $47.5%$47.5% (half of $95%$95%) will lie between the mean and $2$2 standard deviations above the mean:

We can use a similar trick to conclude that $34%$34% (half of $68%$68%) lies between $1$1 standard deviation below the mean, and the mean itself.

If we add these approximations together, we conclude that $81.5%$81.5% (which is $34%+47.5%$34%+47.5%) of data values lie between $1$1 standard deviation below and $2$2 standard deviations above the mean.

Play around with this applet by moving the endpoints of the shaded region. You will see the percentage of data values lying between the endpoints, and can reveal the percentages of each piece with the toggle:

The estimated proportion tells us the probability that our data will have a value in the given range.

 

Exploration

Standard deviation is a measure of spread that we can apply to everyday contexts. For example, let's say the mean score in a test was $67$67 and the standard deviation was $7$7 marks. This means that:

• a person with a standard score of $1$1 (that is, $1$1 standard deviation above the mean) would have received a mark of $74$74 (as this is $67+7$67+7).
• a person with a standard score of $-2$−2 (that is, $2$2 standard deviations below the mean) would have received a mark of $53$53 (as this is $67-2\times7$67−2×7).

If we are told that the scores were approximately normally distributed, we could go one step further and determine the percentage of students who scored between $53$53 and $74$74.

The number of students that score between $2$2 standard deviations of the mean would be $95%$95%. The normal distribution is symmetric, so half of $95%$95% of students scored between the mean and two standard deviations below. In other words, $47.5%$47.5% of students scored between $53$53 and $67$67.

Using the same reasoning, we know that half of $68%$68% of students scored between the mean and $1$1 standard deviation above. This means that $34%$34% of students scored between $67$67 and $74$74.

So putting the two percentages together, we can say that $\left(47.5+34\right)%=81.5%$(47.5+34)%=81.5% of students scored between $53$53 and $74$74.

 

Practice questions

QUESTION 4

QUESTION 5

QUESTION 6