
Working Mathematically II
Mathematical problems sometimes arise because they are to do with a person's work or with their everyday life. At other times we think about a mathematical problem because it is interesting for its own sake - like a puzzle.
Either way, we have to look for strategies that make the problem easier to understand and that lead to a solution.
Initially, a problem may often be expressed in words. We have to try to understand what the words are saying before we can even begin to work on the mathematics.
An important step in understanding a problem and finding a way to a solution is to draw a diagram. We can draw representations of the problem on paper or, with experience, we can form mental pictures that do the same job.
Another, related, strategy is to use symbols of some kind that capture the essential aspects of the problem and the important relationships, but leave out everything that is not relevant. This can help us to see clearly what has to be done to solve the problem.
The use of symbols in this way is what we are doing when a problem is expressed using algebraic symbols. People sometimes find it hard to believe that algebra was invented to make problem-solving easier but that is exactly what Muhammad ibn Musa al-Khwarizmi, the ninth century originator of the method, had in mind.
Mathematical problems come in many different types and no single technique can be applicable to all of them. Decisions have to be made about how to process any numbers that may appear in the problem. For example, should we use addition, subtraction, multiplication or division in some combination or sequence, or should we just arrange the numbers in order or compare them in some way?
The job of the problem solver is to firstly understand the problem and then to choose the relevant facts and mathematical techniques that will lead to a solution.
Example
A sandwich shop prepares orders for customers from $3$3 types of bread, $7$7 different fillings and $2$2 condiments. A sandwich can have $1$1, $2$2 or $3$3 fillings and $0$0, $1$1 or $2$2 condiments. How many combinations of these ingredients are possible?
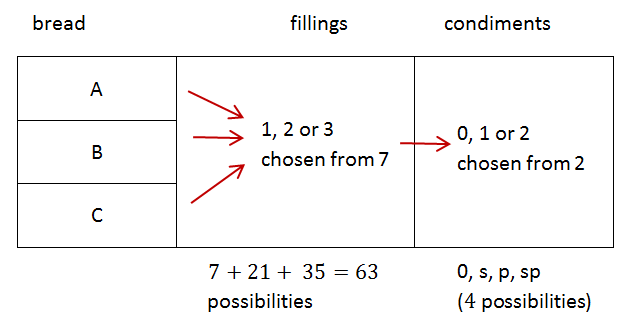
We might begin to understand the problem by making a chart something like the one above. It is easy to list the four possibilities available for the choice of condiments but the possibilities for the fillings is more difficult. Clearly, there are $7$7 ways to choose just one filling. You could make a list using the seven letters a, b, c, d, e, f, g, to count the number of ways of choosing two fillings. There are $21$21 of these two at a time combinations. Similarly, a list of the fillings three at a time would show that there are $35$35 such possibilities. You might be able to find a more sophisticated way of counting the possible combinations.
Since there are $3$3 bread choices and for each of them there are $63$63 filling combinations and for each of these there are $4$4 condiment possibilities, there must be $3\times63\times4=756$3×63×4=756 combinations in total.
Worked Examples
Question 1
The product of two numbers is $18$18 and the sum of the two numbers is $11$11. What are the two numbers?
Write both numbers on the same line separated by a comma.
Question 2
Mohamad owns $2$2 pairs of socks, $4$4 polo shirts and $2$2 trousers. How many different combinations of socks, polo shirts and trousers can he possibly wear?
Question 3
Solar system distances are so great that units other than miles or kilometres are often used. For example, $1$1 astronomical unit (AU) is defined as the average distance between Earth and the Sun, and is equal to $92900000$92900000 miles.
If the distance of Venus from the Sun is $67.2$67.2 million miles, what is its distance from the Sun in astronomical units? Round your answer to 3 decimal places.