Class X

Surface Area of Simple Composite Solids

Lesson

So we know that surface area is the total area of all the faces on a 3D object. We have looked at surface area of prisms and cylinders.

Sometimes though the shape is a composite solid, (made up of a combination of other solids). These are all composite solids.

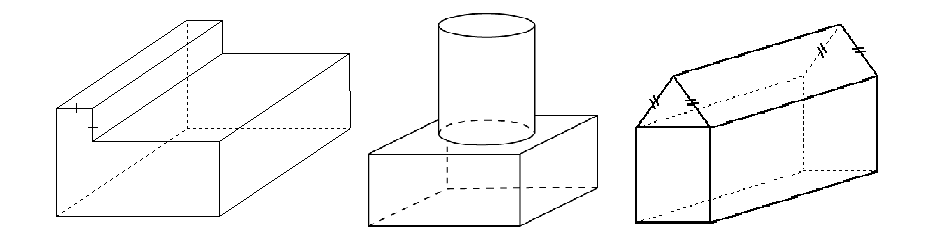
To find the surface area of composite solids we need to be able to visualise the different shapes that make up the various surfaces. Once we have identified the different faces and shapes, calculate the areas of each face and add them up separately.

Don't forget to subtract faces which are not on the surface, like the circle where the cylinder sits on the rectangular prism in the middle image above.

Other common mistakes to be careful about are to not forget faces you may not be able to see in the diagram like those at the back or on the bottom.

Here are some worked examples.

Examples

Question 1

Find the surface area of the figure shown.

Question 2

Find the surface area of the prism shown.

Round your answer to two decimal places.

A solid composed of a square prism and a horizontal cylinder with its bottom half cut off attached on the top rectangular face of the square prism. The solid is oriented such that one of the square faces of the square prism and one of the semicircular faces of the horizontal cylinder are cut in half face front. The diameter of the cylinder is the top side of the square face of the square prism. A side of the square face of the square prism measures $5$ m. The width of the whole solid measures $10$ m.

Outcomes

10.M.SAV.1

Problems on finding surface areas and volumes of combinations of any two of the following: cubes, cuboids, spheres, hemispheres and right circular cylinders/cones. Frustum of a cone.