Find the Equation of a Cubic Function

 

The graphical forms of a cubic

We are often required to derive a function's equation from information contained in its graph. The detective work necessitates a good understanding of the essential form of the basic functions, and then using the particular features of the curve (locations of intercepts, zeros, centres etc) to uniquely identify the function.

When dealing with cubic curves, we are looking for one of the following shapes:

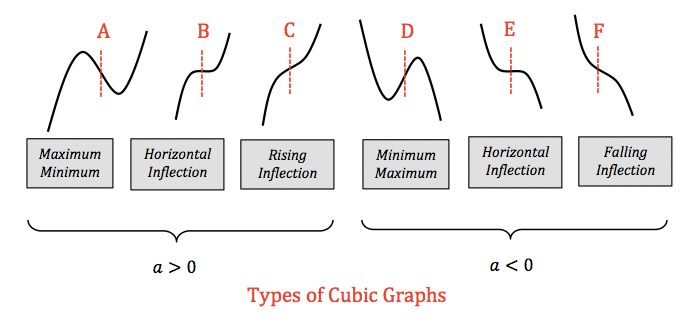
The six graphs are broken into two sets according to whether the coefficient of the leading term $a$a is positive or negative. 

Each graph shows a vertical red dashed line cutting across the cubic's central point. This point could be half way between two local extrema (graphs A and D), or one of two types of inflection - a horizontal inflection (graphs B and E) or a rising or falling inflection (graphs C and F).

The general equation of the cubic is given as $y=ax^3+bx^2+cx+d$y=ax3+bx2+cx+d. A nice way to classify the six graphs is based on calculus techniques and involves the quantity $b^2-3ac$b2−3ac. The above six graphs fall into three categories shown here in the table.

Graph	Features	Test
A,D	A maximum and a minimum turning point	$b^2-3ac>0$b2−3ac>0
B,E	A horizontal inflection	$b^2-3ac=0$b2−3ac=0
C,F	A rising or falling Inflection	$b^2-3ac<0$b2−3ac<0

The function forms of a cubic

Two of the graphs (Graphs B and E) have immediately recognisable function forms given by $y=a\left(x-h\right)^3+k$y=a(x−h)3+k.  It can be thought of as the translated form of the base function $y=ax^3$y=ax3 with the horizontal inflection found at $\left(h,k\right)$(h,k). 

Two other graphs (Graphs C and F) are also immediately recognisable in that they have forms that contain exactly one linear factor and an irreducible quadratic factor, and this means the graph can only ever cross the x - axis once. 

The other two graphs (Graphs A and D) have function forms that very much depend on the relative position of the $x$x - axis. In regard to these graphs, think about this diagram showing different possibilities:

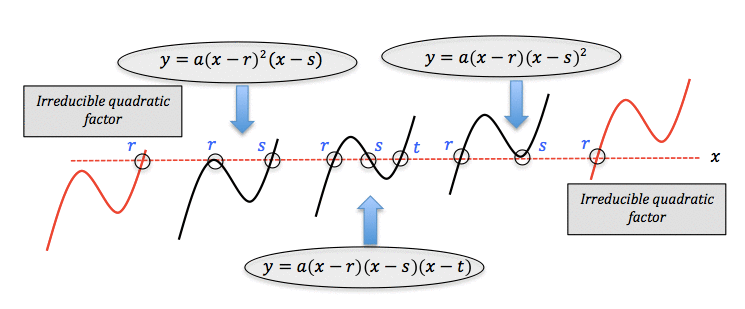
It shows the five essentially different positions of graph A relative to the $x$x - axis. The same sort of scenario would apply to functions with a negative leading coefficient $a$a.

Note that the two red curves in the outside positions show graph type A with the single zero $x=r$x=r. They correspond to cubic functions of the form $y=a\left(x-r\right)\left(x^2+px+q\right)$y=a(x−r)(x2+px+q) where the quadratic expression is irreducible. This means it's unable to be broken into two simpler linear factors.

What this also means is that it is difficult to find the equations of the two outside graphs. It can be done, however, we need to be given at least four pieces of information on each graph before we can determine their equations. For example if we knew the coordinates of four points on any one of the graphs, we could use simultaneous equations to find its unknown coefficients.     

The three inner positions of graph type A  can be completely factored into linear factors as shown by the equations attached to each curve in the above diagram. 

Finding the cubic's equation

We are now in a position to determine the equation of a graphed cubic, and we will give some examples to illustrate the techniques. 

Example 1

Clearly this is a cubic graph of type B - a horizontal inflection at $\left(3,1\right)$(3,1) and a $y$y-intercept of $-53$−53.

The function therefore has the form $y=a\left(x-3\right)^3+1$y=a(x−3)3+1 and all that we need to do is to determine the positive coefficient $a$a. We will use the known point $\left(0,-53\right)$(0,−53) to determine it.

Thus we can write $-53=a\left(-3\right)^3+1$−53=a(−3)3+1 and solve for $a$a as follows: 

$-53$−53	$=$=	$a\left(-3\right)^3+1$a(−3)3+1
$-54$−54	$=$=	$-27a$−27a
$a$a	$=$=	$2$2

Therefore the equation of the graphed cubic is $y=2\left(x-3\right)^3+1$y=2(x−3)3+1.

Example 2

Here we see a cubic graph of type D with three $x$x intercepts of $1,4$1,4 and $6$6 and another known point $\left(7,-9\right)$(7,−9).  

The functions form is thus $y=a\left(x-1\right)\left(x-4\right)\left(x-6\right)$y=a(x−1)(x−4)(x−6) with $a<0$a<0.

The known point provides the clue to $a$a. It must satisfy the equation and so we can write $-9=a\left(7-1\right)\left(7-4\right)\left(7-6\right)$−9=a(7−1)(7−4)(7−6), or, simplified, $-9=18a$−9=18a. This means $a=-\frac{1}{2}$a=−12​ and the function depicted must be $y=-\frac{1}{2}\left(x-1\right)\left(x-4\right)\left(x-6\right)$y=−12​(x−1)(x−4)(x−6). 

Example 3:

This curve has a double zero at $x=-6$x=−6 and a single zero at $x=0$x=0. Its equation is of the form $y=ax\left(x+6\right)^2$y=ax(x+6)2 (Check this!). 

It looks like $\left(-4,-16\right)$(−4,−16) is the central point of inflection, but we have no way of knowing this yet.

What we do know is that $\left(-4,-16\right)$(−4,−16) is on the curve, and so must satisfy the curve's equation. Thus we have $-16=a\left(-4\right)\left(-4+6\right)^2$−16=a(−4)(−4+6)2. Simplifying, we have $-16=-16a$−16=−16a, and thus $a=1$a=1.

Hence the cubic equation we are looking for is given by $y=x\left(x+6\right)^2$y=x(x+6)2.

Note that from earlier work we know that the central point lies on the vertical line $x=\mu$x=μ  where $\mu$μ is the average of the zeros. The zeros for this cubic are $-6,-6$−6,−6 and $0$0 and their average is $-4$−4, so the given point $\left(-4,-16\right)$(−4,−16) is in fact the inflection point of the function.

More Worked Examples

QUESTION 1

QUESTION 2

QUESTION 3