A person's pulse rate is normally given as a number of beats per minute. However, when measuring someone's pulse, a medical practitioner usually does not want to count the number of heart beats over a full minute.
Instead, it is more convenient to count the number of beats in a shorter time, say, $15$15 seconds and then to use this number to predict how many beats there would be in a full minute.
Thus, if $14$14 beats were counted in a period of $15$15 seconds, we would argue that since there are $4$4 lots of $15$15 seconds in a minute we must multiply the $14$14 by $4$4 and get $56$56, the number of beats that would occur per minute.
Another approach would be to measure with a stopwatch the time taken for a fixed number of heart beats, say $10$10, and to use this time measurement to work out the rate per minute. This is illustrated in the following example.
Example
After some physical exertion, a person's heart was found to beat $10$10 times in $4.5$4.5 seconds. What is their heart rate in beats per minute?
Method 1:
Ask how many periods of $4.5$4.5 seconds are there in $60$60 seconds. The number of beats per minute will be $10$10 times this number. So, we calculate $\frac{60}{4.5}\times10=133$604.5×10=133 beats per minute.
Method 2:
Ask how many beats there would be in $1$1 second, or in this case, what fraction of a beat would there be in $1$1 second. The number of beats per minute will be $60$60 times this number. We calculate $\frac{10}{4.5}\times60=133$104.5×60=133, as before.
You should notice that the calculation contains the same steps in both methods. The difference is in the way the problem is conceptualised.
Quantities like heart beats per minute, kilometres per hour, litres per second and so on, are called rates. They involve measurements of and comparisons between two kinds of quantity.
When there is a comparison between two similar kinds of quantity we speak of a ratio. Thus, blood pressure is more like a ratio. It is expressed as what looks like a ratio. It involves two pressure measurements written in the form of a fraction.
The two measurements of pressure are called the systolic and the diastolic pressures. They correspond respectively to the pressures at and between heartbeats.
$\text{blood pressure}=\frac{\text{systolic pressure}}{\text{diastolic pressure}}$blood pressure=systolic pressurediastolic pressure
However, unlike other ratios, we do not normally cancel the blood pressure ratio down to lowest terms because the actual systolic and diastolic measurements are each important and these would be lost if any cancelling down occurred in the fraction.
Pressures are measured in millimetres of mercury (mm.Hg) in the medical context because of a historical method of measuring pressure in terms of the height of a column of mercury the pressure would support. In physics, the pressure is defined as force per unit area so that a pressure of $1$1 pascal is equal to a force of $1$1 Newton per square metre. Several other units of pressure measurement are currently used for various purposes.
So the pressure is a rate - force per unit area - but blood pressure is, roughly speaking, a ratio formed by two rates - the systolic and diastolic pressures.
Worked Examples
Question 1
A pulse was recorded beating at $11$11 times per $10$10 seconds. How many beats per minute is this?
Question 2
Answer the following questions based on the graph.

According to the graph, a fit person has a resting heart rate of around:
$40$40-$60$60 beats per minute.
A$60$60-$80$80 beats per minute.
BThe peak heart rate of an unfit person appears to be:
$120$120 beats per minute.
A$100$100 beats per minute.
B$80$80 beats per minute.
C$140$140 beats per minute.
D
Question 3
Here is a graph showing the normal blood pressure ranges for different aged people. Answer the following questions based on the graph.
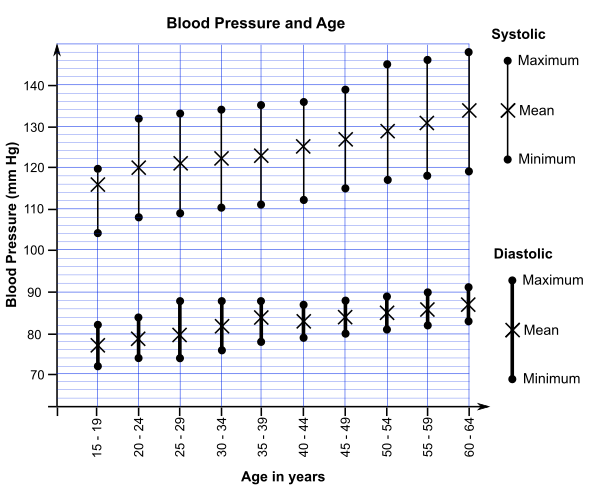
What is the normal diastolic blood pressure range for the age group $30$30 to $34$34?
$\editable{}$ to $\editable{}$ mm Hg
What is the mean systolic blood pressure for this same age group?
$\editable{}$ mm Hg
What is the normal diastolic blood pressure range for the age range $55$55 to $59$59?
$\editable{}$ to $\editable{}$ mm Hg
What is the mean systolic blood pressure for this same age range?
$\editable{}$ mm Hg
Which of the following statements is true, based on the graph?
At any age, the diastolic blood pressure is always lower than the systolic blood pressure.
AA $25$25 year old person will always have lower blood pressure than a $45$45 year old person.
BThe mean diastolic blood pressure increases with age for every age group.
CThe diastolic blood pressure range is larger in older age groups.
D
