Graph Parabolas

Reviewing the four basic forms

In a previous unit of work, we met the four basic orientations of the parabolas' whose axes are lined up with the coordinate axes. We reproduce the diagram here:

 

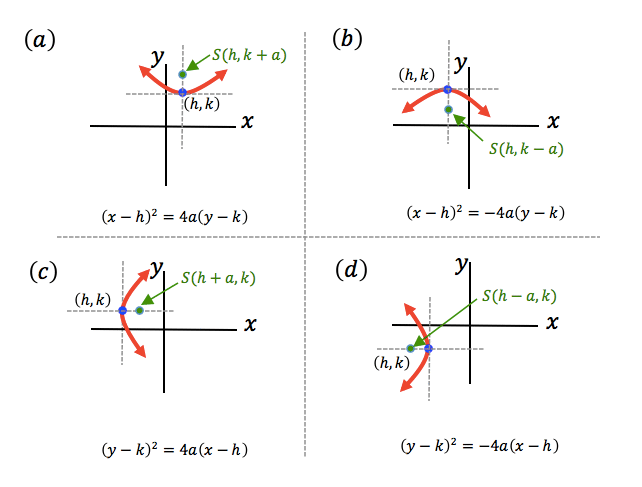
In this unit of work we look at these and other forms of the equation with a view to capture certain identifying information about the parabolas they are associated with in order to be able to graph them. 

a word on letters 

Sometimes in mathematics (and this is one of them), we find that when we generalise particular forms of the equation, we choose letters for coefficients that unfortunately have different meanings when generalising other forms of the same equation. 

For example, the general form of the parabola, used in most mathematics books, is given by $y=ax^2+bx+c$y=ax2+bx+c. We know that the letter $c$c is the $y$y intercept (by putting $x=0$x=0, $y=a\times0^2+b\times0+c=c$y=a×02+b×0+c=c), and the axis of symmetry is the line given by $x=-\frac{b}{2a}$x=−b2a​. We also know that if $a>0$a>0, the parabola opens upward.

However, again in most text books and as we have seen in other units, the same parabola is often expressed in the vertex form given as $\left(x-h\right)^2=4a\left(y-k\right)$(x−h)2=4a(y−k) where the vertex is located at $\left(h,k\right)$(h,k) and where this time $a$a is the focal length of the parabola.

We have to understand that using $\left(x-h\right)^2=4a\left(y-k\right)$(x−h)2=4a(y−k) or perhaps $\left(x-h\right)^2=4c\left(y-k\right)$(x−h)2=4c(y−k) or even $\left(x-m\right)^2=4p\left(y-n\right)$(x−m)2=4p(y−n) really doesn't change the essential information contained in the form. Of course most mathematicians most of the time agree on the use of certain letters in these equations, but we need to be always mindful of the context in which they are used.

MorPH from form to form

We also have important algebra rules that allow us to rearrange an equation from one for to the other. Take for example the parabola given by $y=2x^2-20x+32$y=2x2−20x+32.

We know immediately that the parabola opens upward, the $y$y intercept is $32$32, and the axis of symmetry is given by $x=-\frac{b}{2a}=\frac{20}{4}=5$x=−b2a​=204​=5. 

We might then try to rearrange the equation to a factored form. Of course sometimes a parabola has no $x$x intercepts and therefore the equation will not be factorable over the reals. Let's see what happens;

$y$y	$=$=	$2x^2-20x+32$2x2−20x+32
	$=$=	$2\left(x^2-10x+16\right)$2(x2−10x+16)
$\therefore$∴     $y$y	$=$=	$2\left(x-8\right)\left(x-2\right)$2(x−8)(x−2)

Our endeavours have paid off. From this form we recognise the two $x$x intercepts of $x=2$x=2 and $x=8$x=8, and the average of these accords with our earlier discovery that the axis of symmetry is the line $x=5$x=5.

Finally we can rearrange the original equation, by completing the square, to vertex form as follows:

$y$y	$=$=	$2x^2-20x+32$2x2−20x+32
$y$y	$=$=	$2\left(x^2-10x+16\right)$2(x2−10x+16)
$y$y	$=$=	$2\left(x^2-10x+25-9\right)$2(x2−10x+25−9)
$y$y	$=$=	$2\left[\left(x-5\right)^2-9\right]$2[(x−5)2−9]
$y$y	$=$=	$2\left(x-5\right)^2-18$2(x−5)2−18
$\therefore$∴     $\left(x-5\right)^2$(x−5)2	$=$=	$\frac{1}{2}\left(y+18\right)$12​(y+18)

More information is captured with this form. It has the form depicted by graph (a) above. The vertex has coordinates $\left(5,-18\right)$(5,−18) and the focal length is found by solving $4a=\frac{1}{2}$4a=12​. With $a=\frac{1}{8}$a=18​ this also means that the focus is located at $\left(5,-17\frac{7}{8}\right)$(5,−1778​) and the directrix is the line $x=-18\frac{1}{8}$x=−1818​. Both the focus and the directrix lie very close to the parabola. 

Here is the graph:

 

The same strategies can apply to any of the four orientations of parabolas shown at the top of the page. We can complete squares on $x$x (or $y$y if need be) and find vertices and foci . We can use the formula $x=-\frac{b}{2a}$x=−b2a​ (or in the case of a parabola that opens sideways  $y=-\frac{b}{2a}$y=−b2a​) to find the axis of symmetry.

We will discuss a few examples:

Example 1 

For $y=\frac{1}{9}x^2$y=19​x2, express in the form $x^2=4ay$x2=4ay, and write down the coordinates of the vertex and focus, and the equation of the directrix.

You'll note that the smaller the coefficient of $x^2$x2, the larger the focal length. When the coefficient is small, the parabola widens and the focus and directrix move away from each other.

 Here, with $x^2=9y$x2=9y, we have $a=2\frac{1}{2}$a=212​, and so with the vertex at the origin and the parabola opening upwards, the focus has coordinates $\left(0,2\frac{1}{2}\right)$(0,212​) and the directrix is given by the line $y=-2\frac{1}{2}$y=−212​. It is easy to sketch.

Example 2

For the parabola given by $x=\frac{1}{8}y^2+1$x=18​y2+1, find the position of the vertex, focus and directrix. Which way does the parabola open up, and are there any $y$y intercepts?

A quick rearrangement shows that $y^2=8\left(x-1\right)$y2=8(x−1), and this means that we are dealing with a right opening parabola of the form given by (c) in the diagram at the top of the page.

Since $4a=8$4a=8 we have $a=2$a=2. The vertex is positioned at $\left(1,0\right)$(1,0), and because it is lying sideways, the focus has coordinates $\left(3,0\right)$(3,0), and the directrix has the equation $x=-1$x=−1. There are no $y$y intercepts, because, using the form given in the question, there can be no real solution to $-1=\frac{1}{8}y^2$−1=18​y2. With a vertex at $\left(1,0\right)$(1,0) and a curve opening to the right, there is no possibility of a $y$y intercept. A sketch is easy to construct.

Example 3

Find the coordinates of the focus for the parabola given by $x=y^2-8y+12$x=y2−8y+12.

We first complete the square on $y$y:

$x$x	$=$=	$y^2-8y+12$y2−8y+12
	$=$=	$y^2-8y+16-4$y2−8y+16−4
	$=$=	$\left(y-4\right)^2-4$(y−4)2−4
$\therefore$∴     $\left(y-4\right)^2$(y−4)2	$=$=	$\left(x+4\right)$(x+4)

This implies that $4a=1$4a=1 and therefore $a=\frac{1}{4}$a=14​. The parabola opens to the right, and because the vertex is given by $\left(-4,4\right)$(−4,4), the coordinates of the focus are $\left(-3\frac{3}{4},-4\right)$(−334​,−4). 

Example 4

Example 5

Example 6