Write Equations of Parabolas

Given certain identifying information, it is possible to construct the equation of a parabola. It becomes fairly straightforward when the axes of the parabola are parallel to the coordinate axes. 

In such cases, there are four basic orientations to consider as shown in this diagram. In each of the parabolas, the focus $S$S is positioned on the axis of symmetry of the parabola and on the same side that the parabola is opening to.

 

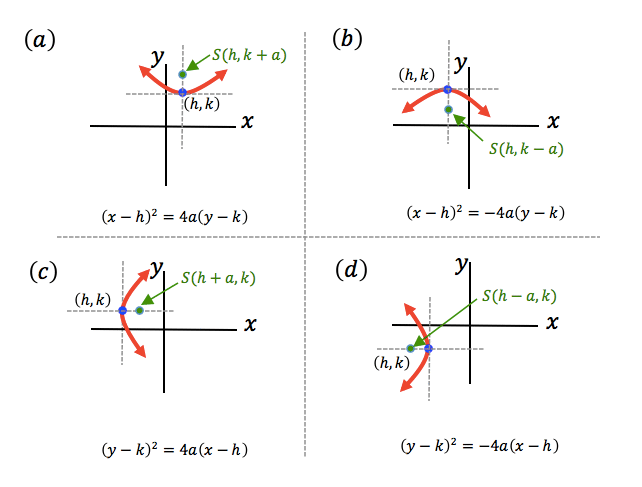
Consider the following examples where we can use this diagram to construct the equation.

Example 1 

A parabola has its vertex at the origin. It's focus lies on the y-axis, $5$5 units directly below the vertex.

Diagram (b) above shows a focus directly below the vertex. By putting $\left(h,k\right)=\left(0,0\right)$(h,k)=(0,0), we see that the basic form of the equation becomes $x^2=-4ay$x2=−4ay. We also know that the focal length $a=5$a=5, and because the vertex is at the origin, it must mean that the coordinates of the focus are $\left(0,-5\right)$(0,−5).

The equation becomes $x^2=-20y$x2=−20y., and the directrix is the line $y=-5$y=−5.

Example 2

A particular parabola with a vertex at the origin opens upward and passes through the point $\left(-12,3\right)$(−12,3). 

From the diagram, this parabola is clearly given by $x^2=4ay$x2=4ay. Points on the curve satisfy the equation, so we know that $\left(-12\right)^2=4a\left(3\right)$(−12)2=4a(3), and when solved we establish that the focal length is $12$12.

The equation becomes $x^2=48y$x2=48y, the directrix is the line $y=-12$y=−12, and the focus is at $\left(0,12\right)$(0,12). 

Example 3

Find the equation of a parabola with its vertex at $\left(-4,3\right)$(−4,3) and its directrix at $x=-9$x=−9. 

Since the directrix is the line $x=-9$x=−9 and the vertex is at $\left(-4,3\right)$(−4,3), then the focal length must be $5$5 (in other words the gap between $x=-9$x=−9 and $x=-4$x=−4 is clearly $5$5). 

The directrix is on the opposite side of the curve to the focus, so the focus must have the coordinates $\left(1,3\right)$(1,3). 

Clearly the curve opens outward to the right, and this means (referring to the diagram) that the equation has the form $\left(y-h\right)^2=4a\left(x-k\right)$(y−h)2=4a(x−k). 

The equation is therefore $\left(y-3\right)^2=20\left(x+4\right)$(y−3)2=20(x+4).  

 

example 4

A certain parabola has a horizontal axis of symmetry, a vertex at $\left(2,6\right)$(2,6) and passes through the point $\left(-30,-10\right)$(−30,−10). Find the equation of the parabola.

If the parabola passes through the point $\left(-30,-10\right)$(−30,−10), and the vertex is at $\left(2,6\right)$(2,6), then quite clearly, the parabola is opening up to the left. Hence the correct form to choose is form (d) above given by $\left(y-h\right)^2=-4a\left(x-k\right)$(y−h)2=−4a(x−k). 

Hence the equation becomes $\left(y-6\right)^2=-4a\left(x-2\right)$(y−6)2=−4a(x−2). Again, the point $\left(-30,-10\right)$(−30,−10) satisfies the equation, so we need to solve $\left(-10-6\right)^2=-4a\left(-30-2\right)$(−10−6)2=−4a(−30−2) for $a$a.

Thus:

$\left(-16\right)^2$(−16)2	$=$=	$-4a\left(-32\right)$−4a(−32)
$\left(-16\right)^2$(−16)2	$=$=	$128a$128a
$256$256	$=$=	$128a$128a
$\therefore$∴     $a$a	$=$=	$2$2

The equation of the parabola is therefore $\left(y-6\right)^2=-8\left(x-2\right)$(y−6)2=−8(x−2). Its directrix is the vertical line $x=4$x=4 and the focus has the coordinates $\left(0,6\right)$(0,6).   

 

More Examples

Question 1

Question 2

Question 3