To form an equation of a sine or cosine curve from a graph or given information we need to identify the key features of the cyclic function. Once we have the key features identified we can construct the general form of the equations. Namely:
$f\left(x\right)=a\sin\left(bx-c\right)+d$f(x)=asin(bx−c)+d or $f\left(x\right)=a\cos\left(bx-c\right)+d$f(x)=acos(bx−c)+d
| vertical translation | Find the central point of the function, an imaginary line that the function appears to oscillate around. This is the value of $d$d in the general form of the equation. |
| amplitude | The maximum deviation of the function from the central line. This is the value of $a$a in the general form of the equation. |
| period | The length of one complete cycle. The value of the period is equal to $\frac{2\pi}{b}$2πb. We will use this and some algebra to determine the value of $b$b for the general form of the equation. |
| phase shift | Is there a horizontal translation? If so, then there is a phase shift of the function. The phase shift is equal to $\frac{c}{b}$cb. We will use this, and some algebra and the value of $b$b that we determined from the period to find the value of $c$c for the general form of the equation. |
| reflection | If there is a reflection, then the sine curve starts at the central line but decreases, and the cosine curve starts at the minimum and increases. If there is a reflection, then you make the value of $a$a negative. |
Examples
Example 1
Find the equation of the cosine curve that has undergone the following transformations:
- a vertical translation of $4$4 units up
- a vertical dilation by a multiple of $2$2
- a horizontal translation resulting in a phase shift of $\frac{\pi}{4}$π4 units left
- a horizontal dilation by a multiple of $\frac{1}{2}$12
Think:
The general form of the cosine equation is $f\left(x\right)=a\cos\left(bx-c\right)+d$f(x)=acos(bx−c)+d, for which we need values of $a,b,c$a,b,c and $d$d. If we start by considering the $5$5 types of transformations and the values that they relate to we can determine the values of $a,b,c$a,b,c and $d$d for this equation.
Do:
| vertical translation | we are told $4$4 units up | $d=4$d=4 |
| amplitude | we are told a vertical dilation of a multiple of $2$2 | $a=2$a=2 |
| period | we are told a horizontal dilation by a multiple of $\frac{1}{2}$12, so the period changes from being $2\pi$2π in length to just half that which is $\pi$π in length. | $\frac{2\pi}{b}=\pi$2πb=π so $b=2$b=2 |
| phase shift | we are told a horizontal translation by $\frac{\pi}{4}$π4 units left. we also know that phase shift =$\frac{c}{b}$cb (remember that left is negative) | $\frac{c}{b}=-\frac{\pi}{4}$cb=−π4 so $c=-\frac{\pi}{2}$c=−π2 |
| reflection | there is no reflection, so the value of $a$a will be positive |
Putting all this together into the general form we determine the equation.
$f\left(x\right)=a\cos\left(bx-c\right)+d$f(x)=acos(bx−c)+d becomes $f\left(x\right)=2\cos\left(2x+\frac{\pi}{2}\right)+4$f(x)=2cos(2x+π2)+4
Example 2
Find the equation of the sine curve in the graph below.

Think:
The general form of the sine equation is $f\left(x\right)=a\sin\left(bx-c\right)+d$f(x)=asin(bx−c)+d, for which we need values of $a,b,c$a,b,c and $d$d. I start by marking on the diagram all the detail I can see that will help me. Namely, the period, central line, amplitude, any noticable reflection or any phaseshift.
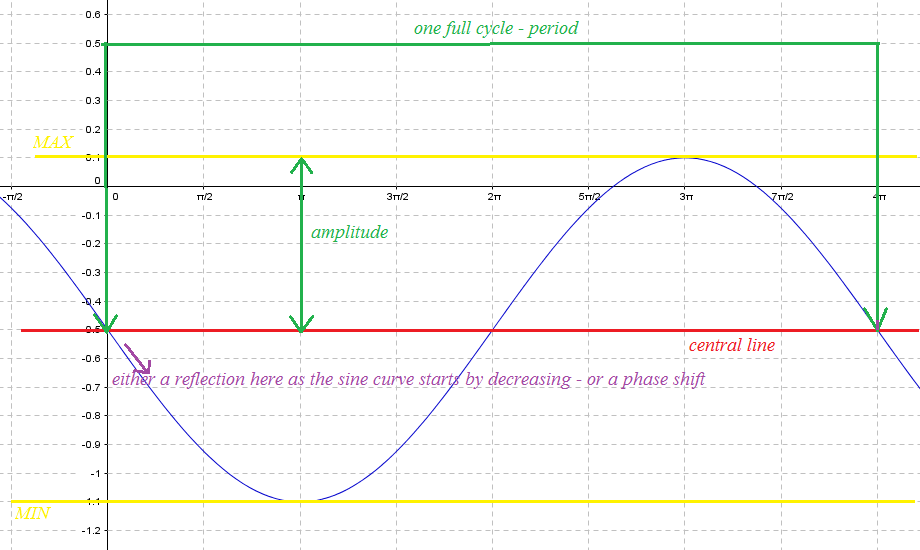
When finding equations we can sometimes get confused about whether there is a reflection or a phaseshift. Unless we are given specific information to check which applies, we will assume a phase shift has occurred.
Do:
| vertical translation | we can see in the diagram this is $0.5$0.5 units down. | $d=-0.5$d=−0.5 |
| amplitude | we can see the amplitude is $0.6$0.6 units | $a=0.6$a=0.6 |
| period | we can see on the graph that one full cycle takes $4\pi$4π | $\frac{2\pi}{b}=4\pi$2πb=4π so $b=\frac{1}{2}$b=12 |
| phase shift | As a sine curve normally starts by increasing from the central line, we can see that there is a phase shift here of $2\pi$2π units. | $\frac{c}{b}=2\pi$cb=2π so $\frac{c}{\frac{1}{2}}=2\pi$c12=2πso $c=\pi$c=π |
| reflection | As we have assume a phase shift, we say there is no reflection. |
Putting all this together into the general form we determine the equation.
$f\left(x\right)=a\sin\left(bx-c\right)+d$f(x)=asin(bx−c)+d becomes $f\left(x\right)=0.6\sin\left(\frac{x}{2}+\pi\right)-0.5$f(x)=0.6sin(x2+π)−0.5
More Worked Examples
QUESTION 1
Determine the equation of the graphed function given that it is of the form $y=a\sin bx$y=asinbx or $y=a\cos bx$y=acosbx, where $b$b is positive.
QUESTION 2
Determine the equation of the graphed function given that it is of the form $y=c+\cos x$y=c+cosx or $y=c+\sin x$y=c+sinx.
QUESTION 3
Determine the equation of the graphed function given that it is of the form $y=\cos\left(x-c\right)$y=cos(x−c), where $c$c is the least positive value.