Angles and Lengths in Quadrilaterals Revision

All quadrilaterals

• Sum of exterior angles of a polygon is $360$360°
• Angle sum of a quadrilateral is $360$360°

 

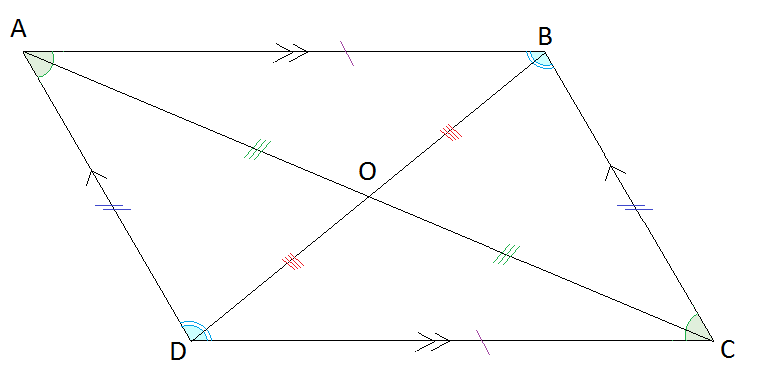
Parallelogram Opposite angles in a parallelogram are equal   Opposite sides in a parallelogram are parallel   Opposite sides in a parallelogram are equal   Diagonals of a parallelogram bisect each other $BO=DO$BO=DO and $AO=CO$AO=CO)
	Rectangle Opposite sides in a rectangle are equalOpposite sides in a rectangle are parallel   Angles in a rectangle are equal to 90°    Diagonals of a rectangle bisect each other ($BO=AO$BO=AO= $DO=CO$DO=CO )   Diagonals in a rectangle are equal ($BD=CA$BD=CA)
Square All sides of a square are equalAll angles in a square are equal to 90°    Opposite sides in a square are parallel   Diagonals of a square are perpendicular to each other (cross at 90°)   Diagonals of a square bisect the angles at the vertices (makes them 45°)   Diagonals of a square bisect each other ($BO=DO$BO=DO= $AO=CO$AO=CO )   Diagonals of a square are equal ($AC=BD$AC=BD)
	Rhombus Opposite angles of a rhombus are equalOpposite sides in a rhombus are parallel   All sides of a rhombus are equal   Diagonals of a rhombus bisect each other at 90 degrees ($BO=DO$BO=DO and$AO=CO$AO=CO)   Diagonals of a rhombus bisect corner angles. i.e. $\angle OAB=\angle OAD$∠OAB=∠OAD,  $\angle OCD=\angle OCB$∠OCD=∠OCB,  $\angle OBC=\angle OBA$∠OBC=∠OBA and $\angle ODC=\angle ODA$∠ODC=∠ODA   Diagonals of a rhombus bisect each other   ($BO=DO$BO=DO and $AO=CO$AO=CO)
Kite 1 pair of opposite equal angles2 pairs of equal adjacent sides   The longest diagonal of a kite bisects the angles through which it passes. i.e. $\angle BAO=\angle DAO$∠BAO=∠DAO and $\angle COD=\angle COB$∠COD=∠COB    Diagonals of a kite are perpendicular to each other.   The longest diagonal of a kite bisects the shorter diagonal ($BO=OD$BO=OD)
	Trapezium >1 pair of opposite parallel sides
Isosceles Trapezium Diagonals of an isosceles trapezium (trapezoid) are equal ($DB=AC$DB=AC)1 pair of opposite parallel sides

 

 

 

Now that you know about 6 different types of quadrilaterals. Move the points (vertices) around and see how many different quadrilaterals you can find in the interactive below.  (Watch this video if you would like to see this interactive in action -Watch video)

The following applet will allow you to manipulate different quadrilaterals using the blue points and see the properties appear with regards to the diagonals.  Watch video

Worked Examples

Question 1

Question 2

Question 3

 

 

Solving problems

When solving angle problems in geometry one of the most important components is the reasoning (or rules) you use to solve the problem.  You will mostly be required in geometry problems to not only complete the mathematics associated with calculating angle or side lengths but also to state the reasons you have used.  Read through each of these rules and see if you can describe why and draw a picture to represent it.

Properties of quadrilaterals

All Quadrilaterals

Angle sum of an n-sided polygon is (n-2)[x]180
Sum of exterior angles of a polygon is 360°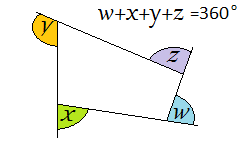
Angle sum of a quadrilateral is 360°

 

Parallelogram

• Opposite sides in a parallelogram are parallel
• Opposite angles in a parallelogram are equal
• Opposite sides in a parallelogram are equal
• Diagonals of a parallelogram bisect each other 

 

Rectangle

• Opposite sides in a rectangle are parallel
• Opposite sides in a rectangle are equal
• Diagonals of a rectangle bisect each other 
• Diagonals in a rectangle are equal 

 

Square

• All sides of a square are equal
• Opposite sides in a square are parallel
• Diagonals of a square are perpendicular to each other (cross at 90°)
• Diagonals of a square bisect the angles at the vertices (makes them 45°)
• Diagonals of a square bisect each other 
• Diagonals of a square are equal 

 

Rhombus

• Opposite angles of a rhombus are equal
• Opposite sides in a rhombus are parallel
• All sides of a rhombus are equal
• Diagonals of a rhombus bisect each other at 90 degrees 
• Diagonals of a rhombus bisect corner angles 
• Diagonals of a rhombus bisect each other

 

Trapezium

• An isosceles trapezium (trapezoid) has 2 pairs of adjacent angles equal
• A trapezium (trapezoid) has one pair of opposite sides parallel
• An isosceles trapezium (trapezoid) has one pair of opposites sides equal
• Diagonals of an isosceles trapezium (trapezoid) are equal

 

Kite

• A kite has 2 pairs of adjacent sides equal
• A kite has 1 pair of opposite angles equal
• The longest diagonal of a kite bisects the angles through which it passes 
• Diagonals of a kite are perpendicular to each other 
• The longest diagonal of a kite bisects the shorter diagonal

 

A summary of the geometrical properties of angles and angles on parallel lines can be found here.  

A summary of the geometrical properties of triangles can be found here.  

Worked Examples

QUESTION 1

QUESTION 2

QUESTION 3