Graph quadratics in turning point form
We have already seen the turning point form of a quadratic, and how using the method of completing the square can transform any standard quadratic into turning point form (sometimes called square form).
Turning point form immediately tells us the turning point (or vertex), and if we know:
- The concavity (positive or negative),
- The vertex, and
- One other point
Then we can accurately graph a quadratic function presented to us in turning point form:
$y=a\left(x-h\right)^2+k$y=a(x−h)2+k
Shape
As we know, the concavity is a measure of how narrow or how wide the parabola is, and whether there is a reflection or not. That is:
- If $a<0$a<0 then the quadratic is concave down
- If $a>0$a>0 then the quadratic is concave up
The larger the magnitude of $a$a, the narrower the curve.

Vertex
The vertex is the turning point of the parabola and it lies on the axis of symmetry. From the equation $y=a\left(x-h\right)^2+k$y=a(x−h)2+k, we can see that the turning point has the coordinates $\left(h,k\right)$(h,k).
If we compare $y=x^2$y=x2, whose vertex is at $\left(0,0\right)$(0,0), to $y=a\left(x-h\right)^2+k$y=a(x−h)2+k, then we can think of the second equation and its graph as a transformation of $y=x^2$y=x2:
- $h$h represents the number of units that $y=x^2$y=x2 is translated horizontally, and
- $k$k represents the number of units that $y=x^2$y=x2 is translated vertically.
Note that with the direction of the horizontal translation:
- If the equation is $y=\left(x+1\right)^2-3$y=(x+1)2−3, the horizontal translation is $1$1 unit to the left.
- If the equation is $y=\left(x-1\right)^2-3$y=(x−1)2−3, the horizontal translation is $1$1 unit to the right.
Graphing the quadratic
Let's look at the quadratic $y=-2\left(x-3\right)^2-3$y=−2(x−3)2−3.
Shape - We can see that the quadratic will be concave down because $a=-2$a=−2, which is less than $0$0.
Vertex - We can see that the quadratic will have turning point at $\left(3,-3\right)$(3,−3).
With just these two pieces of information we can roughly sketch the curve.
But many parabolas are concave down and have a vertex at $\left(3,-3\right)$(3,−3). To be able to graph the parabola more accurately with the correct amount of steepness, we need another piece of information: We need another point on the graph.
A common point to use would be the $y$y-intercept, and we can find this by setting $x$x to $0$0.
| $y$y | $=$= | $-2\left(x-3\right)^2-3$−2(x−3)2−3 |
| $=$= | $-2\left(0-3\right)^2-3$−2(0−3)2−3 | |
| $=$= | $-2\times\left(-3\right)^2-3$−2×(−3)2−3 | |
| $=$= | $-2\times9-3$−2×9−3 | |
| $=$= | $-18-3$−18−3 | |
| $=$= | $-21$−21 |
$x$x-intercept occurs where the value of $y$y is $0$0
$y$y-intercept occurs where the value of $x$x is $0$0
Now we can sketch the curve. Using vertex $\left(3,-3\right)$(3,−3), the concave down shape and a $y$y-intercept of $-21$−21:
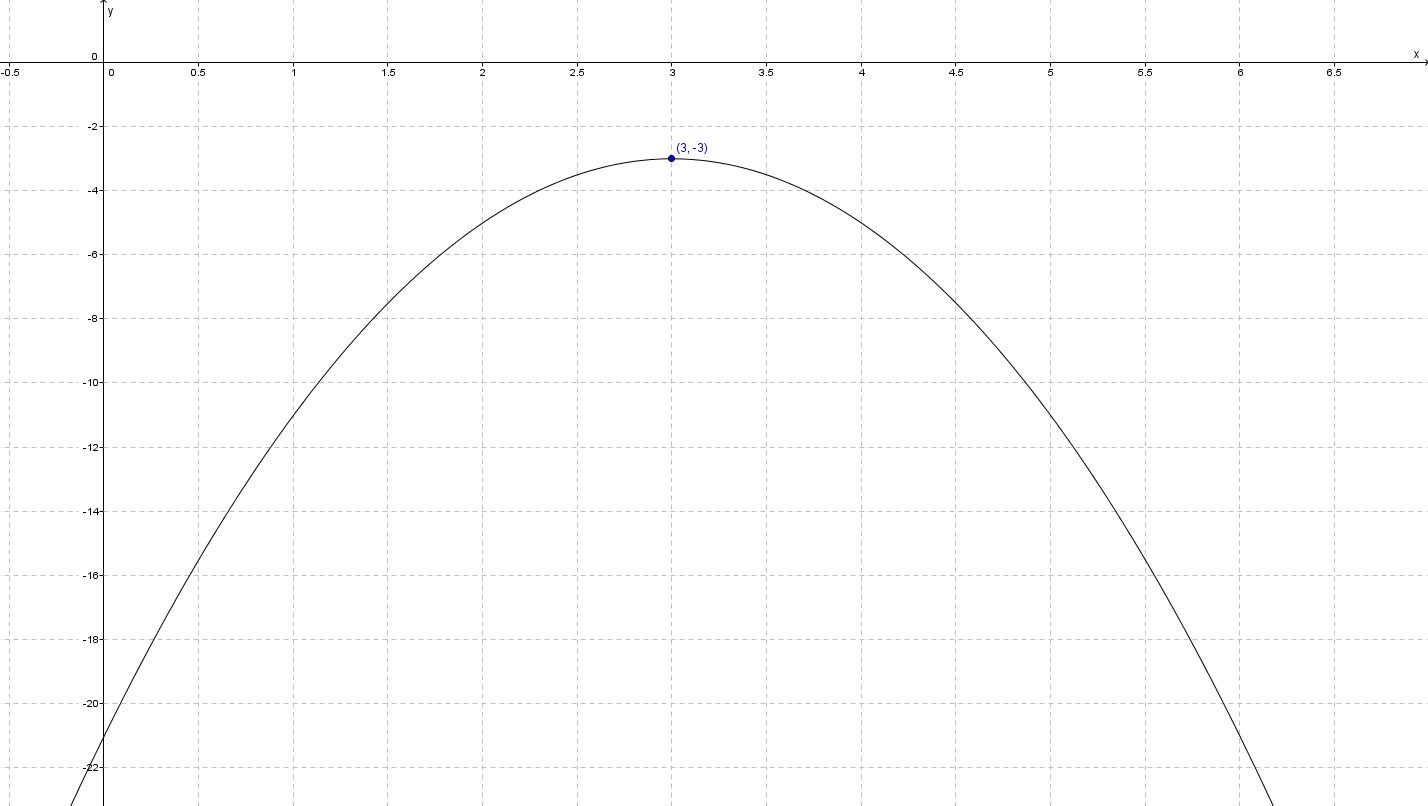
As a hand drawn sketch, this shows all the information we need to sketch the quadratic.

Examples
Question 1
Consider the function $y=\left(x+6\right)^2$y=(x+6)2. Complete the table of values. What is the minimum value of $y$y? What is the $x$x value that corresponds to the minimum $y$y value? Hence state the vertex of the parabola. Vertex $=$=$\left(\editable{},\editable{}\right)$(,) How many units to the left has $y=x^2$y=x2 been translated? $\editable{}$ units Translate the parabola $y=x^2$y=x2 to sketch $y=\left(x+6\right)^2$y=(x+6)2.
$x$x
$-8$−8
$-7$−7
$-6$−6
$-5$−5
$-4$−4
$y$y
$\editable{}$
$\editable{}$
$\editable{}$
$\editable{}$
$\editable{}$
Question 2
Consider the parabola $y=\left(x-2\right)^2-5$y=(x−2)2−5. Which successive transformations turn $y=x^2$y=x2 into $y=\left(x-2\right)^2-5$y=(x−2)2−5? Horizontal shift $2$2 units to the right and vertical shift $5$5 units down Vertical shift $2$2 units down and horizontal shift $5$5 units to the right Horizontal shift $2$2 units to the left and vertical shift $5$5 units up Vertical shift $2$2 units up and horizontal shift $5$5 units to the right Hence state the vertex of the curve. Graph the curve. What is the axis of symmetry?
Question 3
A parabola of the form $y=\left(x-h\right)^2+k$y=(x−h)2+k is symmetrical about the line $x=2$x=2, and its vertex lies $6$6 units below the $x$x-axis. Determine the equation of the parabola. Graph the parabola.