Total surface area
Surface area is the total area of all the faces on a 3D object.
$\text{Surface area of a prism }=\text{Sum of areas of all faces}$Surface area of a prism =Sum of areas of all faces
Surface area of prisms
A prism has two parallel end pieces which are congruent (exactly the same).
It also has a number of faces that join the two end pieces together.
For example, this triangular prism has $2$2 triangular end pieces and then $3$3 faces. This shape would have a net that looks like the following.

The surface area of this shape will be the sum of the area of all the faces. This is the same as the total area of the net containing $2$2 triangle pieces and $3$3 rectangle pieces.
The three rectangles have dimensions equal to the lengths of the sides of the triangle and width equal to the height of the prism.
This interactive shows how to unfold prisms.
Practice question
Question 1
Find the surface area of the triangular prism shown.

Cylinders
The surface of a cylinder is made up of circular faces on the top and bottom and a rectangular face that wraps around the curved surface of the cylinder, as shown in the diagram below.
 |
 |
| A cylinder and its corresponding net | |
One of the dimensions on this rectangle will be the height of the cylinder. The other dimension of the rectangle will be the circumference of the circular base, which can be found using the formula $2\pi r$2πr, where $r$r is the radius of the cylinder.
 |
 |
| Dimensions of the curved face of a cylinder | |
We can explore this further in the applet below. As the circle rolls, notice how its circumference is equal to the length of the rectangle. Drag the point on the circle, or let the animation roll the circle for you.
Type 1 - cylinder with two closed ends
The surface area of a closed cylinder is the sum of the area of the two circular end faces and the area of the rectangle formed by unrolling the curved face.
$SA=2\times\pi r^2+2\pi r\times h$SA=2×πr2+2πr×h
Type 2 - cylinder with one closed end (like a circular swimming pool)
$SA=\pi r^2+2\pi r\times h$SA=πr2+2πr×h
Type 3 - cylinder with two open ends (like a tunnel)
$SA=2\pi r\times h$SA=2πr×h
If we are given the diameter of the cylinder, d then we first need to calculate the radius, $r=\frac{d}{2}$r=d2, to use the surface area formula.
Worked example
Find the surface area of the cylinder below.
Think: The cylinder will unravel into a rectangle with the dimensions $2\pi r$2πr and $h$h and two circles with radius of $r$r. We can use the formula $SA=2\times\pi r^2+2\pi r\times h$SA=2×πr2+2πr×h to determine the surface area.
Do: The radius of the cylinder is $3$3 m2 and the height is $7$7 m2. Let's substitute these values into the formula:
| $SA$SA | $=$= | $2\times\pi r^2+2\pi r\times h$2×πr2+2πr×h |
The formula for the surface area of a cylinder |
| $=$= | $2\times\pi\times3^2+2\pi\times3\times7$2×π×32+2π×3×7 |
Substitute the values into the formula |
|
| $=$= | $60\pi$60π |
Evaluate the multiplication |
|
| $=$= | $188.50$188.50 m2 |
Find the approximate answer, rounding to two decimal places |
Practice questions
Question 2
Find the surface area of the cylinder shown.
Give your answer to the nearest two decimal places.

Question 3
A cylindrical can of radius $7$7 cm and height $10$10 cm is open at one end. What is the external surface area of the can correct to two decimal places?
Question 4
The diagram shows a water trough in the shape of a half cylinder.

Find the surface area of the outside of this water trough.
Round your answer to two decimal places.
Pyramids
A pyramid can be made in the following way. Use any polygon as a base. There can be square bases, triangular bases or even hexagonal bases. Then, connect every vertex of the base to an apex point above the base, and you have a pyramid.

Square and rectangular based pyramids, are the most common you will come across in mathematics, but also in the real world.
In the interactive below, notice that the slope height corresponds to the height of the 2D triangle, which is used in calculating surface area.
$\text{Surface area of right pyramid }=\text{Area of base }+\text{Area of triangles }$Surface area of right pyramid =Area of base +Area of triangles
Practice question
Question 5
Find the surface area of the square pyramid shown. Include all faces in your calculations.

Spheres
A solid three-dimensional circular object is a sphere.

Archimedes showed that the surface area of the circular component of the cylinder wrapping the circle, has area $2\pi r\times2r=4\pi r^2$2πr×2r=4πr2 and that this area is the same value for the surface area of a sphere.
$\text{Surface area of a sphere }=4\pi r^2$Surface area of a sphere =4πr2
Practice question
Question 6
Find the surface area of the sphere shown.
Round your answer to two decimal places.
Composite solids
Sometimes, the shape is a composite solid, it is made up of a combination of other solids. These are some examples of composite solids.
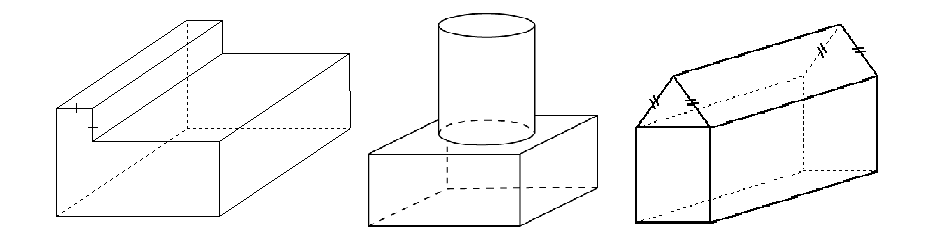
To find the surface area of composite solids, it is necessary to be able to visualise the different shapes that make up the various surfaces. Once these are identified (the different faces and shapes), then calculate the areas of each face and add them up separately.
Don't forget to subtract faces which are not on the surface, like the circle where the cylinder sits on the rectangular prism in the middle image above.
Practice questions
Question 7
In the diagram, the roof has a height of $3$3 metres. Find the surface area of the figure shown,
Round your answer to two decimal places.

Question 8
We wish to find the surface area of the given solid.

What is the surface area of the faces as seen from the top view?
What is the surface area of the faces as seen from the left side view?
What is the surface area of the faces as seen from the front view?
Therefore, what is the total surface area, including all faces of the solid?


