3. Transformations & Congruence
Investigation: Using transformations to rearrange a room
Lesson
Objectives
- Identify practical applications of translations, reflections and dilation
- Use dilations and translations to rearrange a room
Vocabulary
- Translation: a transformation in which an image is formed by moving every point on the preimage the same distance in the same direction.
Translation
- Reflection: a transformation in which an image is formed by reflecting the preimage over a line called the line of reflection (all corresponding points in the image and preimage are equidistant from the line of reflection)

Reflections
- Dilation: a transformation in which an image is formed by stretching or compressing the preimage by a scale factor to create a similar figure

Dilation
- Scale drawing: A drawing which represents a space and the objects in it proportionally but often on a much smaller scale. For example, the picture below is of a field, but is much, much smaller than the actual field, but it is proportional.

Scale drawing of a field
Materials
- Measuring tape
- Two pieces of grid paper
- One piece of blank paper
- Ruler
- Pens, pencils, colored pencils
Group discussion questions
- What actions in your every day life might be represented with translations? reflections? dilations?
- Find two different examples of pieces of art or artists which use at least one of the transformations we have seen. Do you think most people would notice the mathematics behind the art? Explain.
Activity
Sometimes, we might feel the need for change or maybe we are adding a new piece of furniture, but we will rearrange a room. Some rooms will have lots of space, while others might be very tight for space.
We want to create a scale drawing of a room and then create a few different options for the set-up of the room.
Procedure
- Choose a room in your house or your school. On the blank piece of paper, do a rough sketch of the room.
- Measure every length of the room using the measuring tape and put the measurements on your rough sketch. This does not need to be to scale, it is just to record the measurements.

Rough sketch
- Based on your measurements, create a scale drawing of this room on your first piece of grid paper. You can use your proportional reasoning to create find an appropriate scale factor based on the size of your room and the size of your paper. For example, 2 ft = 1 in.

Scale drawing of room
- Measure at least four pieces of furniture or other large objects in the room and mark them down on your rough sketch.
- Using your second piece of grid paper, cut out a scaled version of each piece of furniture.

Cut outs of furniture
- Set up the room as it is currently set up using your cut-out pieces.

Furniture placed as is
- Create another set up for the room using only translations
- For each piece of furniture, state the transformations which have taken place
- Create another set up for the room using any kind of transformation
-
For each piece of furniture, state the transformations which have taken place
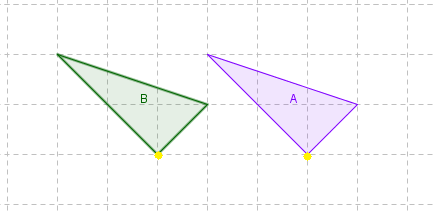
Here is an example of an interactive version of the room. For each piece of furniture, the top point will allow for translations and the bottom point will allow for rotations.
Discussion questions
- When you make a scale drawing, what type of transformation are you performing?
- When you slide a piece of furniture, what type of transformation are you performing?
- When you rotate a piece of furniture 90 \deg, 180 \deg or 270 \deg, what type of transformation are you performing?
- Who do you think might use scale diagrams in this way?
- When might it be worth making a scale diagram instead of just starting to move furniture around? Describe at least three scenarios.