17.10 Solving equations and inequalities graphically (Extended)
Solving equations graphically
Consider the linear equation $2x+4=-6$2x+4=−6. We can immediately start solving it as shown below:
| $2x+4$2x+4 | $=$= | $-6$−6 |
| $2x+4-4$2x+4−4 | $=$= | $-6-4$−6−4 |
| $2x$2x | $=$= | $-10$−10 |
| $\frac{2x}{2}$2x2 | $=$= | $\frac{-10}{2}$−102 |
| $x$x | $=$= | $-5$−5 |
There is often more than one method available to us in mathematics. The graphical method is also available for this question and we can solve $2x+4=-6$2x+4=−6 by treating each side of the equation as a separate function. We then sketch each function and see where they meet. Hence, we sketch the lines $y=2x+4$y=2x+4 and $y=-6$y=−6 and find the $x$x-coordinate of the point of intersection on the $xy$xy-plane.
We can see below that they intersect at the point $\left(-5,-6\right)$(−5,−6). This is consistent with the $x=-5$x=−5 we got when solving algebraically. (Remember, the $y$y value isn't important, as our original question was only in terms of $x$x.)

In general, if we have a linear equation like $ax+b=cx+d$ax+b=cx+d, then we can check the solution by sketching graphs of $y=ax+b$y=ax+b and $y=cx+d$y=cx+d.
There will usually be one solution, but if the lines are parallel there will be zero solutions and if the lines are collinear there will be an infinite number of solutions.
Solving linear inequalities graphically
Similar to linear equations, we can also make a connection between linear inequalities and graphs of lines. The key difference is that instead of just looking for where they intersect, we are interested in finding what range of $x$x-values one line is above or below the other.
Let's consider the inequality $3x-5>-5x+11$3x−5>−5x+11. On first reading, we can see we are finding $x$x values that make the left-hand side larger than the right-hand side. Graphically, this means we are seeking the values of $x$x for which the line $y=3x-5$y=3x−5 is greater than, or above, $y=-5x+11$y=−5x+11.
We can sketch graphs of both lines like this:
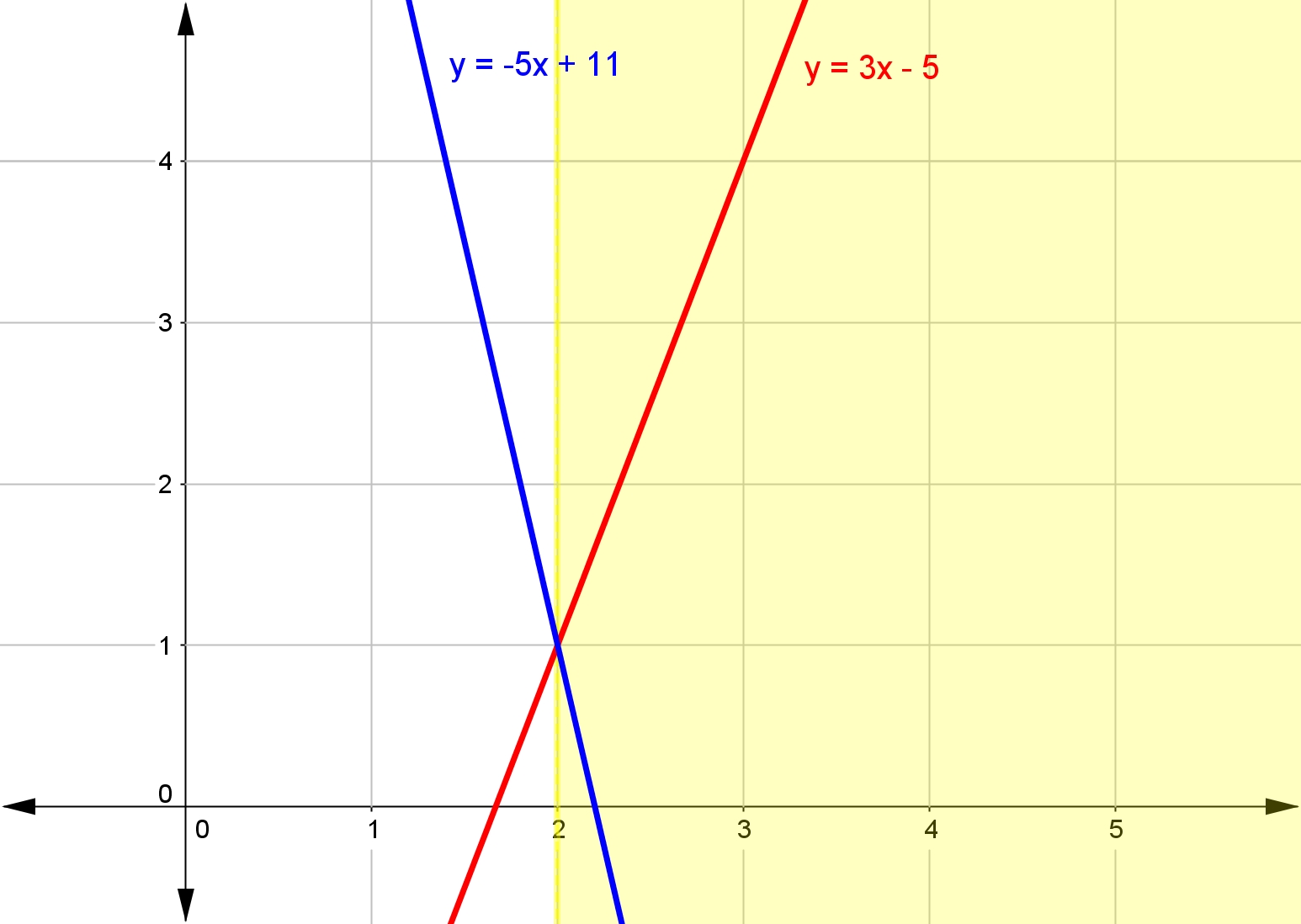
When is the line $y=3x-5$y=3x−5 above the line $y=-5x+11$y=−5x+11? It is clear that this occurs for $x>2$x>2.
Of course, we also have our algebraic skills to solve these questions, which means we are able to check our solution.
| $3x-5$3x−5 | $>$> | $-5x+11$−5x+11 | |
| $8x-5$8x−5 | $>$> | $11$11 | Add $5x$5x to both sides |
| $8x$8x | $>$> | $16$16 | Add $5$5 to both sides |
| $x$x | $>$> | $2$2 | Divide both sides by $8$8. |
Note: You can also graph the functions in the inequality using technology, and then use the generated graph to compare the functions and solve the inequality.
Practice questions
Question 1
Consider the graphs of $y=x+5$y=x+5 and $y=12-x$y=12−x.
Using the graphs, state the solution of the inequality $x+5>12-x$x+5>12−x. Give your answer in interval notation.
Question 2
Consider the graphs of $y=x+6$y=x+6 and $y=x-7$y=x−7.
Which of the following statements is true?
The inequality $x+6\ge x-7$x+6≥x−7 has one solution.
AThe inequality $x+6\ge x-7$x+6≥x−7 has an infinite number of solutions.
BThe inequality $x+6\ge x-7$x+6≥x−7 has no solutions.
C
Solving quadratic inequalities graphically
Now, what if we are given an inequality involving a quadratic, such as $x^2+2x-8>0$x2+2x−8>0? We do not need to know an algebraic method for quadratic inequalities in this course and the graphical method provides an efficient way to find our solutions. We can consider the quadratic function $y=x^2+2x-8$y=x2+2x−8 and find values of $x$x where the function is greater than $0$0.
Consider the sketch of the parabola:

Being asked to find solutions to $x^2+2x-8>0$x2+2x−8>0 is exactly the same as being asked to find values of $x$x at which the graph of $y=x^2+2x-8$y=x2+2x−8 is above the line $y=0$y=0 (the $x$x-axis). In other words, when is $y=x^2+2x-8$y=x2+2x−8 positive?
We can see from the graph here that unlike our previous inequality which provided us with a single range of values for our answer, here, there are two. Our answer has to be written in two parts $x<-4$x<−4 and $x>2$x>2. In interval notation, this could be written as $(-\infty,-4)\cup(2,\infty)$(−∞,−4)∪(2,∞).

What if we had flipped the inequality sign in the question? That is, we were required to solve $x^2+2x-8<0$x2+2x−8<0. Now we are being asked when the graph $y=x^2+2x-8$y=x2+2x−8 is negative. Now our solutions will be $x>-4$x>−4 and $x<2$x<2. This can be written as $-4

Note: You can also graph the functions in the inequality using technology, and then use the generated graph to compare the functions and solve the inequality.
Equivalent inequalities
Suppose we want to solve $\left(x+2\right)^2>2x+12$(x+2)2>2x+12. One approach would be to go through the slightly complicated task of sketching graphs of the parabola on the left and the line on the right to examine when the parabola lies above the line. These graphs would have to be quite accurate to ensure we can clearly identify the points of intersection.
However, we could simplify the inequality first, bringing all of the terms to the left-hand side so that we only need to sketch one graph. Expanding and simplifying, we get:
| $\left(x+2\right)^2$(x+2)2 | $>$> | $2x+12$2x+12 |
| $x^2+4x+4$x2+4x+4 | $>$> | $2x+12$2x+12 |
| $x^2+2x-8$x2+2x−8 | $>$> | $0$0 |
The inequality $\left(x+2\right)^2>2x+12$(x+2)2>2x+12 is thus equivalent to the inequality $x^2+2x-8>0$x2+2x−8>0 from before, and so it will have the same solutions.
Practice questions
Question 3
Consider the graph of $y=f\left(x\right)$y=f(x).
Find the values of $x$x for which $f\left(x\right)=0$f(x)=0. Write both solutions on the same line separated by a comma.
For what values of $x$x is $f\left(x\right)<0$f(x)<0?
Give your answer as an inequality.
For what values of $x$x is $f\left(x\right)>0$f(x)>0?
Give your answer as an inequality.
What is the $x$x-coordinate of the vertex of $f\left(x\right)$f(x)?
Question 4
We want to solve $x^2-2x\le-x+2$x2−2x≤−x+2 by plotting two curves and finding their points of intersection.
First find the $x$x-intercepts of $y=x^2-2x$y=x2−2x.
Find the $y$y-intercept of $y=x^2-2x$y=x2−2x.
Find the $x$x-intercept of $y=-x+2$y=−x+2.
Find the $y$y-intercept of $y=-x+2$y=−x+2.
Hence plot the set of curves for $y=x^2-2x$y=x2−2x and $y=-x+2$y=−x+2.
Loading Graph...Use the graph from the previous part to select the correct option:
The two curves intersect at $\left(-1,3\right)$(−1,3) and $\left(2,0\right)$(2,0), hence the solution to the inequality is$-1\le x\le2$−1≤x≤2.
AThe two curves intersect at $\left(-1,3\right)$(−1,3) and $\left(2,0\right)$(2,0), hence the solution to the inequality is $x\le-1,x\ge2$x≤−1,x≥2.
BThe two curves intersect at $\left(3,-1\right)$(3,−1) and $\left(0,2\right)$(0,2), hence the solution to the inequality is $0\le x\le3$0≤x≤3.
CThe two curves intersect at $\left(3,-1\right)$(3,−1) and $\left(0,2\right)$(0,2), hence the solution to the inequality is $x\le0,x\ge3$x≤0,x≥3.
D
Question 5
To solve the inequality $x\le\frac{x-3}{4}-1$x≤x−34−1, Tracy graphed $y=x-3$y=x−3. What other line would she need to graph to be able to solve the inequality graphically?
$y=4x+1$y=4x+1
A$y=\frac{x-3}{4}-1$y=x−34−1
B$y=x$y=x
C$y=4x+4$y=4x+4
D