Area of a rectangle
A rectangle is a shape with the specific geometric properties of
- opposite sides equal in length
- opposite sides parallel
- sides meet at right angles.
To determine the area of a rectangle we divide the rectangle up into square units and count them.
Here is a rectangle measuring $4$4 units in length and $2$2 units in width.
 |
We divide the rectangle up into its square units and see how many there are |
 |
This rectangle has an area of $8$8 square units. |
To determine the area, we could count the squares, one at a time. Or we could multiply the lengths of the sides because there are $2$2 rows of $4$4, ($2\times4=8$2×4=8) or that there are $4$4 columns of $2$2 ($4\times2=8$4×2=8).
$\text{Area of a rectangle}=\text{length}\times\text{width}$Area of a rectangle=length×width
$A=L\times W$A=L×W
Area of a square
A square is a special kind of rectangle, because it meets all the requirements of a rectangle but has the extra property that all sides are equal length.
To find the area of a square, we use the rectangle rule,
$A=L\times W$A=L×W
Consider this example of a square with sides equal to 4 units:
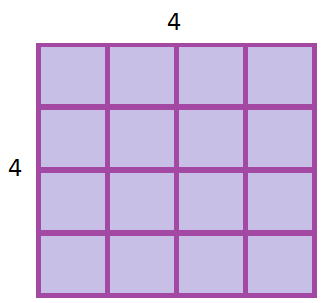 |
Area | $=$= | $4\times4$4×4 | |
| $=$= | $16$16 square units |
As the length and width are the same, the area of a square has a simpler rule that we can use:
$\text{Area of a square}=\text{length}\times\text{length}$Area of a square=length×length
$A=L^2$A=L2
Practice questions
Question 1
Find the area of the attached figure.

Question 2
Find the area of the rectangle shown.

Area of a triangle
The area of any triangle is exactly half of the area of a rectangle that contains it.


Use the applet below to explore this. Drag the vertices of the triangle to change its shape and then slide the slider to see how it turns into a rectangle.
We already know how to find the area of a rectangle using $A=L\times W$A=L×W, and the applet above shows us that the area of a triangle is half of that.
We call the bottom side of the triangle the base. The height is then measured perpendicularly (at right angles) to the base, not along the sloping side.
$\text{Area of a triangle}=\text{half of the area of the rectangle with base and height the same as the triangle}$Area of a triangle=half of the area of the rectangle with base and height the same as the triangle
$\text{Area of a triangle}=\frac{1}{2}\times\text{base}\times\text{height}$Area of a triangle=12×base×height
$A=\frac{1}{2}\times b\times h$A=12×b×h
Practice question
Question 3
Find the area of the triangle shown.

A right-angled triangle is illustrated with its base measures $8$8 m and its height measures $7$7 m. The hypotenuse, side opposite to the right angle indicated by a small box, is not labeled.
Area of a parallelogram
A parallelogram is a shape with the specific geometric properties of
- opposite sides equal in length
- opposite sides parallel
Parallelogram exploration
Use the applet below to explore how a parallelogram can be broken up and turned into a rectangle.
We know that the area of a rectangle is given by $A=L\times W$A=L×W, so we can work out the area of any parallelogram.
We call the bottom side of the parallelogram the base, and we also need the height of the parallelogram. The height must be measured perpendicularly (at right angles) to the base, not along the sloping side, just like for the area of a triangle.
$\text{Area of a parallelogram}=\text{base}\times\text{height}$Area of a parallelogram=base×height
$A=b\times h$A=b×h
The calculation above is exactly the same as the area of a rectangle even though the rule uses different words for the length and width).
Worked example
Example 1
Find the area of the parallelogram shown:

Think: Identify the values for the base and height, then multiply
Do:
| A | $=$= | $b\times h$b×h |
| $=$= | $32\times14$32×14 mm2 | |
| $=$= | $30\times14+2\times14$30×14+2×14 mm2 | |
| $=$= | $420+28$420+28 mm2 | |
| $=$= | $48$48 mm2 |
Practice questions
Question 4
Find the area of the parallelogram shown.

A parallelogram with the horizontal top side labeled 15 cm, suggesting the measurement of its base. A vertical dashed line is drawn beyond the parallelogram, connected to a horizontal dashed line perpendicular to the horizontal top side. This vertical dashed line is labeled 14 cm, suggesting the measurement of the parallelogram's height perpendicular to the base.
Area of a trapezium
A trapezium (sometimes called trapezoid) is a shape with
- One pair of opposite sides that are parallel
All of these are trapeziums:


Trapezium exploration
The interactive below shows that two identical trapeziums can be joined to form a parallelogram. We can use this property to determine the area of a trapezium.
We can see in the diagrams below that two identical trapeziums make a parallelogram with an area:
$\text{Area }=\left(a+b\right)\times h$Area =(a+b)×h.
So a single trapezium must have half of the area of the parallelogram:
$\text{Area }=\frac{1}{2}\times\left(a+b\right)\times h$Area =12×(a+b)×h
|
$\text{Area }=\frac{1}{2}\times\left(a+b\right)\times\text{height }$Area =12×(a+b)×height |
|
$\text{Area of a trapezium}=\frac{1}{2}\times\text{sum of parallel sides}\times\text{height}$Area of a trapezium=12×sum of parallel sides×height
$A=\frac{1}{2}\times\left(a+b\right)\times h$A=12×(a+b)×h
Typically, the letters $a$a and $b$b are used for the length of the parallel sides (it doesn't matter which one is which)
Practice questions
Question 5
Find the area of the trapezium shown.

Question 6
Find the value of $x$x if the area of the trapezium shown is $65$65 cm2.

Start by substituting the given values into the formula for the area of a trapezium.
$A=\frac{1}{2}\left(a+b\right)h$A=12(a+b)h
Areas of kites and rhombuses

A kite is a shape with the specific geometric properties of
- Two pairs of adjacent sides that are equal
- One pair of equal angles
Of course the kite you fly around on a windy day is named after the geometric shape it looks like.
A rhombus is a kite with the additional property that all sides are the same length. (A rhombus also meets all the conditions for a parallelogram.)
Kite exploration
The interactive below shows that a kite can be broken into triangles, which can be used to determine the area.
$\text{Area of a kite }=\frac{1}{2}\times\text{long diagonal }\times\text{short diagonal }$Area of a kite =12×long diagonal ×short diagonal
$A=\frac{1}{2}\times x\times y$A=12×x×y
Typically, the letters $x$x and $y$y are used for the length of the diagonals of the kite (it doesn't matter which way around).
Practice questions
Question 7
Find the area of the kite shown.

Question 8
The area of a kite is $640$640 cm2 and one of the diagonals is $59$59 cm. If the length of the other diagonal is $y$y cm, what is the value of $y$y rounded to two decimal places?
Area of a circle
We already know that area is the space inside a two dimensional shape. We can find the area of a circle, but we will need a special rule.
The following investigation will demonstrate what happens when we unravel sectors of a circle.
When we realign the segments we end up with a parallelogram shape. We can use this to find the area of a circle based on our knowledge that the area of a parallelogram has formula $A=bh$A=bh. The parallelogram formed from a circle has a base length equal to half the circumference and the height is the radius.
$\text{Area of a circle}=\pi r^2$Area of a circle=πr2
The formula above must use the radius. If we instead know the diameter, then we first calculate the radius with the conversion:
$r=\frac{d}{2}$r=d2
Parts of a circle
Half of a circle would have half of the area of the whole circle. Divide the area of a full circle by $2$2.
A quarter-circle would have a quarter of the area of the whole circle. Divide the area of a full circle by $4$4.
Practice questions
Question 9
If the radius of the circle is $5$5 cm, find its area.
Give your answer as an exact value.
Question 10
Consider the sector below.

Calculate the perimeter. Give your answer correct to two decimal places.
Calculate the area. Give your answer correct to two decimal places.
QUESTION 11
A goat is tethered to a corner of a fenced field (shown). The rope is $9$9 m long. What area of the field can the goat graze over?

Give your answer correct to two decimal places.

